|
A common problem is when one has laser with a certain wavelength (color)
but needs some very other wavelength (color). For example: one has a neodymium
laser and wants it to be used for hologram shooting. However most of the photo
materials are not sensitive for infrared light, so the direct use of the Nd
laser is impossible. It might be suggested to use some other laser, e.g.
ruby one or copper vapour one. But this way has its own drawbacks. Copper
laser is a large, complicated and troublesome thing, hard to use. Ruby
laser requires huge amount of pump energy and its beam quality is low. On
the top of this there might be a situation, when You have already got a
neodymium laser, while the other lasers are still to be obtained or created.
It these cases the help comes from non-linear optics. Most probably You
have already heard about "frequency doubling" or "second harmonics generation".
And chinese green laser pointers, that use this frequency doubling widely,
have already become something common.
At the first glance it is very simple: just take a crystal and put there
an invisible beam of neodymium laser. And get the greeny second harmonic out.
In addition the substances for the crystals seem to look affordable too:
dihydrogen phosphates of potassium or ammonium are readily available as
fertilizers. However brief first tries to get the second harmonics do usually
fail. Even a very powerful neodymium laser is unable to give a visible green
spot if its beam was directed to an arbitrary crystal at arbitrary direction.
Whats the reason here? There are usually three of them:
- First of all one needs to have a proper crystal, of course. The crystal
is to have a suitable size, to be transparent enough and to consist of a
material that is able to produce this second harmonic.
- One needs a proper laser too. The "properness" of the laser can be tied
with its peak power in most cases. The rate of conversion (at least at its initial
stage) is proportional to the square of the light intensity (power per unit of
area), so the more powerful laser You have the better. One usually needs a
q-switched laser to perform this task.
- One should also know (at least approximatively) the direction to put the
beam into (the proper angle to set the beam at). Dont expect that You can
put the crystal to the beam, and begin to rotate it randomly in various
directions until it shines with green. Too much degrees of freedom. Too low
probability. With this approach You will exhaust the lifetime of Your laser
or damage the crystal well before You get some green light out of here.
The most simple solution of the problem of crystal presence is to buy a ready
made one over the internet. Use the keywords "SHG laser crystal". Among the
ones available on the market, the most effective ones are KTP and LBO. The
cheapest ones are ADP and KDP. Others will have intermediate performance and
price.
Another way is to grow the crystal all by Yourselves. But it is a separate
story. Look for it near the end of this guide.
The problem of the appropriate laser can be solved by purchasing or creating
one. There are many
Q-switcthed lasers available on the web. Among them are
the famous SSY-1 laser heads, the heads of "Kigre Systems", and many "noname" heads
from chinese laser epilators. If You've got laser crystal and mirrors it is
easy to create the laser all by Yourselves. (See e.g. here) Another deal is
that this problem is most probably not Your case, otherwise You would read
the guide how to create the laser rather than how to convert its beam.
And the third problem - the understanding how to let the beam into the crystal,
will be our business for the next few pages. Generally speaking the non linear
optics is probably the most complicated subject to study in the whole quantum
electronics. One needs to know crystallography, solid state physics, wave
mechanics and so on. Luckily to us there is no need to go deep there in order
to build Your first DIY SHG generator. However a few basic terms are still to
be consumed.
(The bored ones can immediately proceed to the description of experiments,
however if You not only want "to take a look, what did the author do? " but
intend to reproduce it by Yourselves, I insist You to get acknowledged with
the theory, especially taking into account that it was simplified to "no further" here.)
The first and most important term to know is the optical axis of crystal.
It's hard to describe what exactly it is without turning this guide into a
textbook on birefringence, so for now just remember that any birefringent
crystal has an optical axis. (Dont confuse it for crystallographic axes or
other type axes.) The optical axis is not obliged to be parallel to atomic
planes of crystalline lattice or to be parallel to some edges or faces either.
For example in Iceland spar the optical axis is parallel to the direction
from the vertice, where all obtuse angles of its natural faces meet each other to
the another vertice of the same kind. The happy coincidence is that the optical axis is
parallel to the long(est) edge of the crystal in the most interesting for DIYer
crystals of KDP and ADP.
It may be rather difficult to find the direction of the optical axis of
an unknown crystal. The methods being used for it are a bit complicated, and
hard to adopt for home use. To my opinion it's more rational to look at the
natural faceting of the crystal (its habit) and to refer to the
literature (or to the web) to find out how the optical axis is directed
relatively to the natural edges/facets. In case You use a commercial crystal
having been cut in shape of cylinder or box, You should refer to the datasheet
or ask the seller, how was the crystal cut relatively to its optical axis.
Sometimes the direction of the cut is clear from the usage of the crystal.
For example a crystal intended for use in a Pockel's cell (Q-switch) is cut
so that the beam propagates along the optical axis. So this crystal is of
no use as a frequency doubler. (For the reason - refer to below.)
The next important term is the angle of phasematching. Second Harmonic
Generation is a coherent process, so it requires exact synchronization
between phases of radiation being convert (pump wave) and of radiation of
the second harmonic. If the phase shift between the waves wanders randomly,
while the main laser beam and its second harmonic walk through the crystal,
the process of forward energy transfer is compensated by the backward
energy transfer and the resulting conversion can be very very little. Some
media, particularly birefringent crystals, allow to choose such a direction
of beam, that both speeds of light for the first and second harmonics appear
to be identical. In this case one can say that the beam goes in the phase-match
direction.
In the simplest case. when there is only one optical axis, in order to hit
the direction of phasematching, it is enough if the direction of the beam
constitutes a certain angle with the optical axis (Fig 1A). This angle is
called as "phase match angle" and (usually) designated as greek letter "θ".

Figure 1. A) Optical axis direction and phase match angle in KDP and ADP
crystals.
B) Cone of phasematching.
For the most of known crystals, able to be used for SHG, the phase match
angles are known and given in correspondent handbooks. Need to say, however,
that the value of this angle, given in these handbooks, is for the ray inside
the crystal. If one wants to know, how to direct the beam from outside the
crystal, one needs to correct the direction by using the refraction law for
the facet, through which the ray comes in.
Due to the fact that only the angle between the ray and the optical axis
characterizes the direction of the phase match, it actually appears, that
all possible phase match directions form a cone shape (Fig 1B). I.e. if the
beam is unpolarized and walks the same distance inside the crystal, it may
come from any direction chosen from the phase match cone - in any case the
conversion would be the same. Obviously it is related to the beams and
directions inside the crystal. In the outer space the geometrically perfect
cone will be deformed by the refraction in the facets.
Before we can name the exact values of these "magical" angles, for the
exact crystals, we need to know what types of phase match can be. For this
we will need the terms: "ordinary ray" and "extraordinary ray". So suffer
further and get another piece of theory.
-
The ray in (uniaxial) crystal can be ordinary and extraordinary dependently
to the relative orientation of the optical axis of the crystal and the plane
of polarization of the ray. To describe this relative position they use a term
principal plane. The principal plane is the plane that contains the accident
ray itself and also the optical axis of the crystal. Need to note: the optical
axis is not the full determined line. On the contrary it is just a direction,
or a vector with floating beginning point. So one can build the principal plane
in every case when the ray and the optical axis aren't collinear. Otherwise
there are infinitive number of them and the term "principal plane" looses its
sense.
-
The ray having its plane of polarization lying in the principal plane is
called "extraordinary" one and designated by latin letter "e".
-
The ray with the plane of polarization orthogonal to the principal plane is
called "ordinary" one and designated by latin letter "o".

Figure 2. Types of rays in ADP and KDP crystals dependently to the relative
position of their polarization plane and the optical axis of the crystal.
A) Extraordinary ray. Arrows designate that its plane of polarization lies
in the plane of the picture.
B) Ordinary ray. The circles (kinda projections
of arrows) designate that the plane of polarization is orthogonal to the
plane of the picture. The optical axis is shown by dot-dash line as earlier.
NOTE: "In classic" they assume that polarization plane is the plane of
oscillations of the electric field of the light. This convention is taken
in many textbooks. E.g. in [3]. However not all share this opinion. For example
in [4] it is taken that the polarization plane is the plane of magnetic
oscillations. So if one uses a textbook as a handbook: i.e. to get a certain
data or formulae, and does not read it sequentially fro the beginning to the
end, there may appear a quite a mess in the head.
This guide assumes that polarization plane is the plane of electric field
oscillations.
-
Any ray coming into crystal can be considered as one consisting of both
ordinary and extraordinary rays. If the original ray has planar polarization
one can use the "rule of projections". The electric field of the appearing
ordinary ray is equal to the projection of the electric field of the incoming
ray onto the plane, where the polarization of the ordinary beam should be.
The same is true for the extraordinary ray. (Fig 3)

Figure 3. Decomposition of incoming ray into ordinary and extraordinary rays.
The arrows here show not the rays themselves, but the vectors of electric
field in the rays (the same thing, that was sown in figure 2 by short arrowlets).
"E" - electric field of the original ray;
"Eo" - electric field of the appearing ordinary ray;
"Ee" - electric field of the appearing extraordinary ray.
If we designate the angle between the plane of polarization and the optical
axis as "γ", one can get from the picture, that the amplitude of
the electrical field of the extraordinary ray is
Ee=E*cos(γ) and
the amplitude of the electrical field of the ordinary ray is
Eo=E*sin(γ).
E - means the amplitude of the field in the original ray. Let's note that
as intensity of the light is proportional to the square of its field strength,
i.e. I = k*E^2 (where k is some constant), it means that:
Ie + Io = k*Ee^2 + k*Eo^2 = k*E*(cos(γ))^2 + k*E*(sin(γ))^2 = k*E^2 = I
or
Ie + Io = I.
Literally: the intensity of the original ray is exactly equal to the sum of
intensities of the ordinary and extraordinary rays, the original ray was
decomposed into.
Now we've got clarity with ordinary and extraordinary rays. Let's go
further to the types of phase-match. They distinguish only two of them:
- "Type I" - is when some plano polarized wave enters the crystal and
as the result there appears new plano polarized wave, but the plane of
its polarization is orthogonal to the plane of polarization of the
original wave.
- "Type II" - is when two waves enter the crystal. They are both plano polarized.
And their planes of polarization are orthogonal to each other. The appearing
wave of second harmonic has planar polarization too and its plane of
polarization is similar to one of the original waves.
It seems obvious that one needs to put two rays to the entrance of the crystal
for the second type of the phasematch. Their planes of polarization are to be
orthogonal to each other. At the exit there will be the remnants of the two
original rays plus a third ray, having its polarization plane parallel to
the one of an original ray, but differing by the fact that its frequency has been
doubled. (For example for a neodymium laser: two infrared beams are entering
and a new green ray is exiting together with the remnants of the two infrared
rays.)
The phasematching of the first type looks like it needs only one entering ray.
However for the unification it is accepted that there are two rays at the
entrance. Indeed, when having a sole ray, we can always imagine that there are
two of them (say, each has a half intensity) being geometrically coincident
with the original ray.
Hereby it is accepted that in the process of frequency doubling there always
take part two rays of the basic frequency and there appears one ray with the
double frequency.
One can also note, that the fact, that the type II requires two rays, is
also conventional. Looking at the Figure 3, one can see, that it is enough if
the entering ray had its polarization plane at an angle of 45 degrees to the
optical axis. It will then decompose into two rays of equal intensity. To the
ordinary one and the extraordinary one.
There exist "letter" designation of phasematch type. If one remembers that
ordinary ray is signed by letter "o" and extraordinary ray - by letter "e",
then one could easily say that there can be two "type I" phasematches: "ooe"
and "eeo" and two "type II" phasematches: "oee" and "eoo". First two letters
are responsible for the incoming rays and the last letter - for the result
of the interaction.
In one type of crystal there cannot exist both types of phasematch of the
same kind. If in some crystal there exists a type I phasematch of "ooe" kind,
there cannot be type I phasematch of "eeo" kind. This is determined by a
type of the crystal. Crystals can be "positive" (+) and "negative" (-).
The table below shows which kinds of phasematch can exists in different
crystal types:
| Phasematch type V |
Crystal type |
| (-) |
(+) |
| Type I |
ooe |
eeo |
| Type II |
oee |
eoo |
In order for not to turn this guide into a complete schoolbook on crystal
optics, the detailed description of the terms "positive" and "negative"
will be omitted. The methods of determining these "positiveness" and
"negativeness" will be omitted too. I hope it won't be serious loss, because
one can successfully build a frequency doubler without this knowledge.
If You still need the additional information - all the textbooks on optics
and crystallography are at Your service. Here I will only mention that
the overpowering majority of the uniaxial nonlinear crystals, being
used in visible and near visible spectrum, are negative. Particularly
ADP, DADP, KDP, DKDP, LiNbO3, BBO are negative. So for the first time
Yo can take, that "Type I" is "ooe" and "Type II" is "oee".
Even simpler: If Your laser gives unpolarized (or circular polarized) beam,
You only need to remember that each crystal has two phasematch angles.
Two allowed angles between the beam and the optical axis of the crystal.
One of them will be for type I and other for type II.
If Your laser gives planarly polarized beam, You will have to bother not only
with the angle between the beam and the optical axis, but also with the
correct direction of the polarization relatively to the optical axis. For the
type I the plane of polarization should be orthogonal to the optical axis.
For the type II it should be at 45 degrees to the optical axis (see Fig 4)
 
Figure 4. Correct position of polarization plane in relation to the optical
axis of KDP-like crystal. Left is for the type I phasematch, right is for
type II. Laser is shown as a rectangular block. The incoming and outcoming
beams are shown as ribbons, but it does not mean their real geometry. Instead
the plane of the ribbon designates the plane of the polarization. The red color
means incoming (long wave) beam. The green color means the output (short
wave) beam. The picture as a whole is a simplification too. It should not
be taken as a direct guidance to action. At least due to the fact that
in KDP crystal the phasematch angle for type I interaction does not allow
the rays with this angle neither to enter the crystal nor to leave it
through the big natural facets of the crystal.
The dependence of the second harmonic output power on the angle between
the plane of polarization and the optical axis is smooth. In addition
it is weak when this angle is near its optimum. So one does not need to foresee
the means of alignment of the crystal in this direction. It is enough if
laser and crystal were roughly installed so that the plane of polarization
and the optical axis form the needed angle.
Now we've understood the types of phasematch. Its time to show the table
of the phasematch angles for the crystals most affordable (or potentially
affordable) for DIYer.
Table 2. Phasematch parameters (angle, angle tolerance, effective non linearity)
when transforming some wavelengthes into 2-nd harmonic. (The table was
constituted by calculations in SNLO code [5]).
Crystal type,
Phasematch type V |
Wavelength of the beam being doubled |
1064 nm
(YAG:Nd)
silicate Nd:glass |
808 nm
Ti:Al2O3
diode laser |
694 nm
ruby laser |
532 nm
(second
harmonic
of Nd laser) |
450 nm
laser diode
MVL |
KDP
type I (ooe) |
40.9 deg
±2.5 x 2.5 mrad*cm
0.26 pm/V |
44.4 deg
±1.8 x 1.8 mrad*cm
0.3 pm/V |
50.5 deg
±1.5 x 1.5 mrad*cm
0.341 pm/V |
76.0 deg
±2.3 x 2.3 mrad*cm
4.62 pm/V |
no
phasematch
of I type |
KDP
type II (oee) |
58.6 deg
±2.8 x 16.2 mrad*cm
0.337 pm/V |
69.3 deg
±2.8 x 23 mrad*cm
0.26 pm/V |
no
phasematch
of II type |
no
phasematch
of II type |
no
phasematch
of II type |
ADP
type I (ooe) |
41.1 deg
±2.2 x 2.2 mrad*cm
0.374 pm/V |
44.3 deg
±1.6 x 1.6 mrad*cm
0.425 pm/V |
50.3 deg
±1.4 x 1.4 mrad*cm
0.48 pm/V |
76.6 deg
±2.2 x 2.2 mrad*cm
6.64 pm/V |
no
phasematch
of I type |
ADP
type II (oee) |
60.3 deg
±2.6 x 18.2 mrad*cm
0.458 pm/V |
70.6 deg
±2.7 x 26.5 mrad*cm
0.35 pm/V |
no
phasematch
of II type |
no
phasematch
of II type |
no
phasematch
of II type |
DKDP
type I (ooe) |
36.4 deg
±2.8 x 2.8 mrad*cm
0.22 pm/V |
43.3 deg
±1.9 x 1.9 mrad*cm
|
50.8 deg
±1.7 x 1.8 mrad*cm
0.31 pm/V |
84.4 deg
±6.0 x 6.0 mrad*cm
4.3 pm/V |
no
phasematch
of I type |
DKDP
type II (oee) |
53.4 deg
±2.8 x 29.3 mrad*cm
0.339 pm/V |
69.1 deg
+-3.0 x 37 mrad*cm
0.24 pm/V |
no
phasematch
of II type |
no
phasematch
of II type |
no
phasematch
of II type |
DADP
type I (ooe) |
36.9 deg
±2.5 x 2.5 mrad*cm
0.324 pm/V |
43.2 deg
±1.8 x 1.8 mrad*cm
0.387 pm/V |
50.3 deg
±1.5 x 1.5 mrad*cm
0.45 pm/V |
79.6 deg
±3.1 x 3.1 mrad*cm
6.16 pm/V |
no
phasematch
of I type |
DADP
type II (oee) |
54.7 deg
±2.6 x 30.4 mrad*cm
0.48 pm/V |
69.8 deg
±2.8 x 42.1 mrad*cm
0.34 pm/V |
no
phasematch
of II type |
no
phasematch
of II type |
no
phasematch
of II type |
BBO
type I (ooe) |
22.8 deg
±1.1 x 1.1 mrad*cm
2.0 pm/V |
28.9 deg
±0.7 x 0.7 mrad*cm
2.0 pm/V |
34.0 deg
±0.5 x 0.5 mrad*cm
1.96 pm/V |
47.6 deg
±0.4 x 0.4 mrad*cm
1.75 pm/V |
no
phasematch
of I type |
BBO
type II (oee) |
32.9 deg
±0.9 x 37.3 mrad*cm
1.41 pm/V |
42.0 deg
±0.6 x 12 mrad*cm
1.11 pm/V |
50.0 deg
±0.5 x 7.4 mrad*cm
0.82 pm/V |
81.0 deg
±1.3x 10.3 mrad*cm
4.8 pm/V |
no
phasematch
of II type |
LiNbO3
type I (ooe) |
80.9 deg
±3.8 x 3.8 mrad*cm
-4.8 pm/V |
no
phasematch
of I type |
no
phasematch
of I type |
no
phasematch
of I type |
no
phasematch
of I type |
LiNbO3
type II (oee) |
no
phasematch
of II type |
no
phasematch
of II type |
no
phasematch
of II type |
no
phasematch
of II type |
no
phasematch
of II type |
Li2B4O7
type I (ooe) |
31.0 deg
±2.0 x 2.0 mrad*cm
0.065 pm/V |
37.3 deg
±1.4 x 1.4 mrad*cm
0.08 pm/V |
43.7 deg
±1.1 x 1.1 mrad*cm
0.09 pm/V |
65.0 deg
±1.1 x 1.1 mrad*cm
0.13 pm/V |
no
phasematch
of I type |
Li2B4O7
type II (oee) |
no
doubling |
no
doubling |
no
doubling |
no
phasematch
of II type |
no
phasematch
of II type |
Each cell of the table at the cross of the row with the type of crystal
and type of phasematch and the column, correspondent to the wavelength of
incident radiation, contains (sequentially from top to bottom): phasematch
angle (see Fig 1), tolerance of this angle (or one could also take it as
the maximal allowed divergency of the incident beam), and effective non
linearity of the crystal for this particular type of process. If You do not
know what exactly it is, assume that it is a qualitative parameter. The more
it is the lower power of laser radiation is needed to reach the effective
doubling.
The angular tolerance is shown in milliradian multiplied by a centimeter.
What does it mean? If Your crystal has the length of 1 cm (along the beam
propagation) then the angular tolerance will be numerically equal to the
values from the table. If the crystal has the length of 0.5 cm, then - to
doubled value from the table, and if the crystal is 2 cm long, then only
a half of the value given in the table. The longer the crystal - the more
efficient SHG, but the beam can easier come out of the phasematch,
so the tolerance is more strict. It's also apparent, that if generally
two beams are needed to be mixed for the doubling, the angular tolerance
might not be equal for both of the incident beams. Therefore there are two
values of the tolerance, separated by "x" sign. Type I has both incident
rays similar to each other, so the tolerance is equal for both of them. For
the type II the first value of tolerance is for the ordinary ray and the
second one is for the extraordinary ray.
The first 4 rows of the table are of the most interest for DIYers. KDP
and ADP crystals are known to be oftenly grown at home from affordable
resources (from garden fertilizers). BBO and LiNbO3 crystals are wide
spread commercially available ones. DADP and DKDP are commercially available
too - these are deuterated versions of KDP and ADP. Lithium tethraborate
seems to be able to be grown at home provided that one has some persistence.
The best of commercial SHG crystals, such as potassium titanyl phosphate
(KTP) and lithium triborate (LBO) are biaxial ones and therefore they are
out of consideration of this guide. Some words about them will be said at the end of this guide.
Designations of crystal types are common for non-linear optics:
- KDP - potassium dihydrophosphate. Other names: "mono potassium phosphate" or "mono kalium phosphate (MKP)" or potassium phosphate monosubstituted.
Chemical formula: KH2PO4
- DKDP - potassium dideuterium phosphate, other names: "mono kalium phosphate
deuterated", deuterated potassium phosphate monosubstituted.
Chemical formula: KD2PO4
- ADP - ammonium dihydrophosphate. Other names: "mono ammonium phosphate" or
or ammonium phosphate monosubstituted.
Chemical formula: (NH4)H2PO4
- DADP - ammonium dideuterium phosphate, other names: "deuterated mono ammonium
phosphate", deuterated ammonium phosphate monosubstituted.
Chemical formula: (ND4)D2PO4 (dont mess ND4 with neodymium, lol)
- BBO - beta barium borate. BaB2O4. In essence it is acidic salt of barium
and orthoboric acid, but having been dehydrated at melt point.
- Lithium Niobate and Lithium Tetraborate in the table are represented by their
chemical formulas.
The last note for the table: the angles (and angular tolerances too) are
given for the ray inside the crystal. If You need to know the angle to lit
the crystal from the outside, use the correction, given by the refraction law
for the correspondent crystal facet.
EXAMPLE 1: At what angle should come the incident beam of Nd laser through
a natural facet of KDP crystal to obtain frequency doubling of II-nd type?
SOLUTION: Using the table 2 we'll find the phasematch angle for 1064 nm
wavelength for KDP crystal and type II phasematch. It is equal to 58.6 deg.
Therefore the angle of incidence (when the beam hits the facet from the inside
of the crystal) is 90-58.6 = 31.4 deg. (Lemme remind that angle of incidence
in optics is the angle between the ray and the normal to the surface.)
Since the ordinary ray is refracted as usual, we can use Snell's law (fig 5):
sin(θ2)
--------- = 1.48 => θ2 = arcsin(1.48 * sin(31.4°)) = 50.45°
sin(θ1)
1.48 here is the index of refraction of KDP for the ordinary ray having its
wavelength of 1064 nm.
We got that the angle of incidence of the beam outside the crystal should be
equal to 50.45j.
We can also calculate the angle between the ray and the plane of the facet
(outside of the crystal):
θ_out = 90° - θ2 = 39.55°
As You can see, this value is very close to the one, obtained in 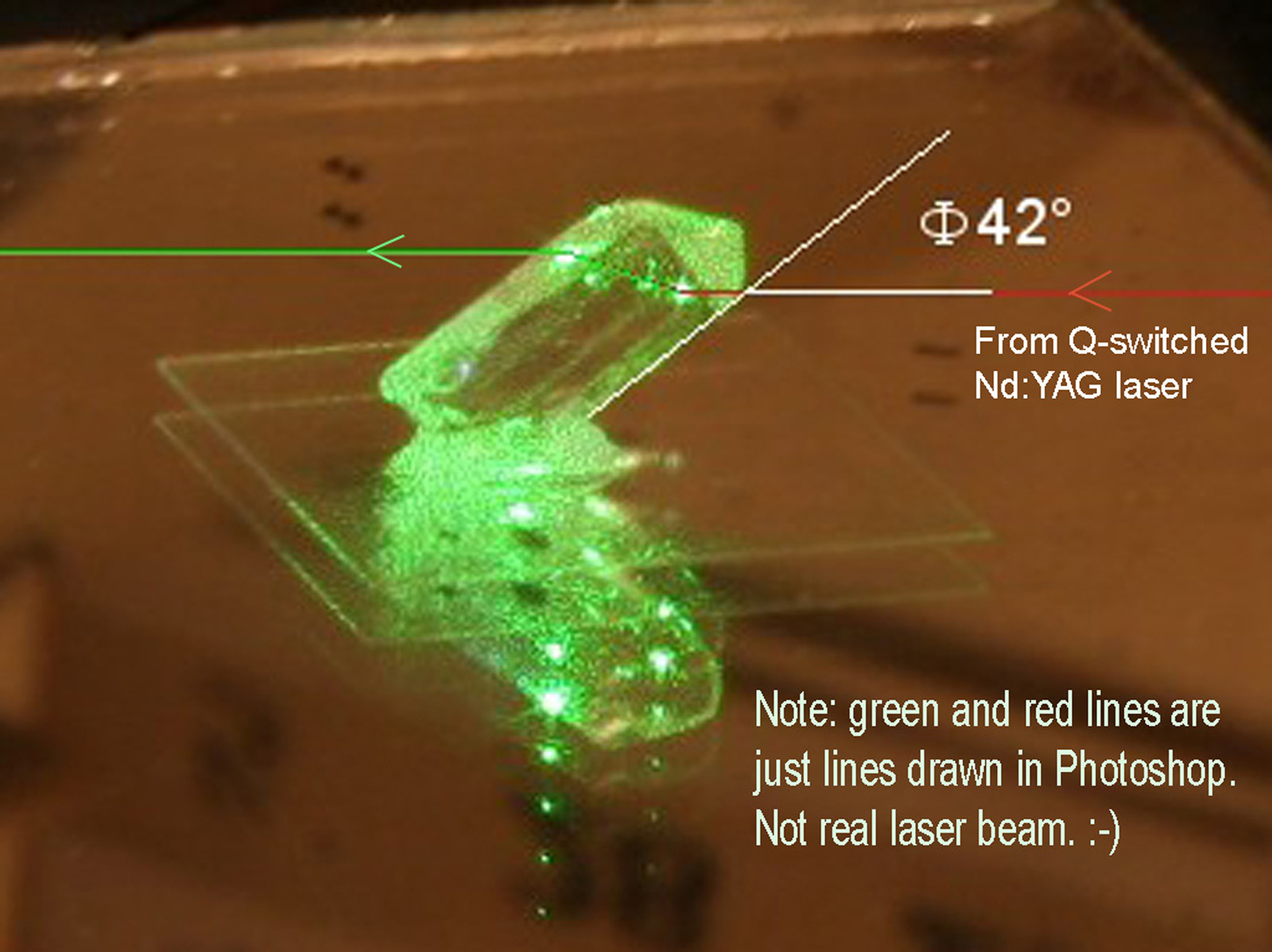 . .
The difference of 2 degrees can be explained by the imperfect orientation
of the facet. The beam had refracted not only in the principal plane, but
in the orthogonal plane also.

Fig 5.
EXAMPLE 2. At what angle should come the incident beam of Nd laser through
a natural facet of KDP crystal to obtain frequency doubling of I-st type?
SOLUTION: Using the table 2 we'll find the phasematch angle for 1064 nm
wavelength for KDP crystal and type I phasematch. It is equal to 40.9 deg.
Thus the angle of incidence onto the natural face (inside) is
90-58.6 = 31.4 deg.
As in the previous case lets use the Snell's law for the ordinary ray to get
the outside angle of incidence (geometry of the task is shown on fig 5).
sin(θ2) = 1.48 * sin(49.1°) = 1.11°
The sine of refraction angle has exceed the unity. Not only it means that
we cannot use arcsin function. It also means that the condition of full
internal reflection has been fulfilled, and any ray coming at this angle
just cannot leave the crystal. And vice versa: any beam coming through the
natural facet into the crystal just cannot be directed at the necessary angle.
Thus: in the naturally faceted KDP crystal one cannot fulfill the conditions
of type I phasematch by directing the beam from outside the crystal. (One
can still try to use those little facets near the pyramidal part of the
crystal, but such an attempts rarely give the desired results in practice.)
Example 2 shows, that for the use of the crystal for the type I doubling
it is not enough to have a naturally faceted crystal. One needs THE CUT.
If one took a diamond saw and cut the crystal at the needed angle to the
optical axis and finally polished the new faces, one could obtain a piece of
crystal, easily allowing to insert a ray at an angle necessary for the Ist type of doubling.
In KDP crystal the IInd type of doubling is possible with a beam entering
through a natural facets. Other crystals aren't obey to follow this rule.
It may be so that for any kind of doubling one needs a cut. Generally the
cuts of crystals are widely spread in the technique and they may be at
different angles dependently on the application (see fig 7).

Figure 6. The scheme of cut stages for the necessary angle. One can see
that if a naturally faceted crystal is used, much of the material goes waste. There exist, however, technologies of growth, allowing crystal to grow
directly in a shape of plane with given orientation relatively to its optical
axis.

Figure 7. Cuts of KDP crystal for different purposes (not to scale).
If You deal with a commercial crystal get ready to that they always use
some cut. In some cases one can use the cut for differing purposes, in
other cases one cannot. For example You have a cut for type I doubler
and You want to use it as type II one. It is quite possible since the
difference in angles is relatively small. However if You have a Pockel's
cell crystal (being usually used as an electrooptic shutter) and You want
to use it as a frequency doubler, in this case the necessary angle of crystal
installation usually does not allow the beam to pass through. Similarly if
one has a doubler for some certain wavelength and wants to use it for
some other wavelength, it is easy if the difference between the wavelengthes
is small. One needs only to turn the crystal slightly to compensate the
angular mismatch. But if the difference between the wavelengthes is large
the required turn angle can be so great, that even when lighting into the
edge of the crystal the conditions are still not fulfilled.
THE PROPERTIES OF FREQUENCY DOUBLING ←
- When the phasematch is exact, the intensity of the second harmonic
rises monotonously as the path of the ray in the crystal grows. It reaches
saturation smoothly and further on the intensity of the second harmonic
does not grow. The characteristic length of path between the beginning
and saturation is in reciprocal proportion to the square root of
the intensity of the incident beam (or as they also say: the pump beam):
Lnl ~ 1/sqrt(Io)
- When there is some phase mismatch (imperfect hit of the phasematch angle
or detuned wavelength or something else) the intensity of the second harmonic
does not grow monotonously with the increasement of the path inside the
crystal. It begins to oscillate. One can observe the forward energy transfer
from the original beam to the beam of second harmonic and backward energy
transfer from the second harmonic to the first one. The higher is the
mismatch, the shorter is the period of the oscillations.
One of the consequences from this property is that if the phase mismatch
is large enough it is almost impossible to manufacture the crystal, that
supports only forward energy transfer. The other important consequence is
that in the process of the angular alignment of the crystal You won't
observe a monotonous grows of the second harmonic power, as the angle comes
closer to the exact match. You will have plenty of local maximums and
minimums instead. And from those ones You will have to choose the best.
- When the path of the ray in the crystal is short, when the energy
transfer is negligible, it is true that: I(SHG)~I(o)^2 - the intensity of
the second harmonic is in square proportion to the pump beam intensity.
The simplest way to explain this is the next: let's consider the process
of doubling as a process of interaction of two pump photons, that disappear
in this process, and give birth to the third photon of doubled frequency.
The rate of any process involving particles-projectiles and particles-targets
is in direct proportion to the number of particles-targets in the unit
of the beam cross-sectional area. It is alsp in the direct proportion to the number
of particles-projectiles in the same area. However in our case both
particles-targets and particles-projectiles are the same photons of the
original (pumping) radiation. It means that the rate of the process is
twice proportional to their density. In other words it is proportional to
the square of their density, or finally, to the square of the intensity of
light. (Attention: in order to make this simple statement to become the
full scale argumentum, one needs to take tons of delicate things into account,
and this will expand the procedure to a page or two. Lets just omit this and
let it be.)
- When the path of the ray in the crystal is large, and the energy transfers
almost completely, it is obvious that I(SHG)~I(o). The intensity of the second
harmonic is directly proportional to the intensity of pumping. The ratio of the
proportionality (or in other words: the effectiveness of the doubling) may
be different dependently on the type of the crystal, beam quality and the
precision of match.
For the industry it is typical to obtain the efficiency of doubling up to
30..50% when the crystal is outside the laser and up to 90% when it is inside
the laser's resonator. Most of the contemporary laser pointers are examples
of lasers with intracavity doubling. However one may expect that in future they
will be forced out by laser pointers having a 520 nm laser diode as a direct
source of the green beam.
In DIY conditions, when one has a small q-switched laser and a home grown
crystal of decent quality one may hope for ~10% for the doubling of near
infrared light into the visible and ~3-5% for turning the visible into UV light.
SHG WITH FOCUSING ←
Since the outcome of the second harmonic in a crystal of given size grows
strongly with the growth of the intensity of the incident beam, one will always
want to increase this intensity. At least by focusing. However there is a pair
of tricky things.
The first (and trivial one): having the laser radiation been focused into
a small spot You can (easily) reach the limit of the light endurance of the
particular crystal. The damage may appear on the surface or either in the
volume. It is obvious that after the appearance of the typical star-shaped
bunch of crackles the crystal becomes unusable.
The second: by focusing the beam You add a certain angle of
divergency/convergency to the beam. It's OK, if this angle is small, but if
it begins to exceed the angular tolerance (see table 2 and notes to the table),
the efficiency of doubling begins to degrade.
The narrow focusing (short focused lense) means smaller spot, higher intensity
in the focal plane, and, at the same time, higher divergency. Note, however,
that the angular tolerance is more strict for longer crystals. It means that
little crystals allow (and even require) more narrow focusing, while for large
crystals the focusing may be excessive. (Thanks goodness if they can cope with the
natural diffraction divergency of the beam.)
Which exactly focal length to choose depends not only on the thickness of Your
crystal, but on the diameter of the laser beam, and on its natural divergency
(on its mode constitution). In my experiments for the crystals with length
of 3..5 mm along the beam path, decent results were obtained with focal lengths
over 50 mm. Changing FL=50 mm to FL=30 mm did not increase yield of second
harmonic. And for FL<30mm the efficiency degraded rapidly.
The wide divergency of the beam being doubled may be useful when searching
for the phasematch angle. If the power of pumping beam is enough and the lights
are dim, one can observe a whole lattice of maximums and minimums on a screen
behind the crystal. Remember though, that the brightness of such a picture is
low. In order to observe it one needs not only to turn lights off, but also
to filter out the light of the laser's lamp.
When focusing one needs to take into account that usually not the visible
light is being focused. Due to the dispersion, the refraction index of the
glass is different and so the focal length. That is why one needs to take an
amendment deltaF for the focal length F:
deltaF -delta(N-1) deltaF -deltaN
---------- = ------------- or -------- = ----------
F (N-1) F (N-1)
where N - the refractive index of the glass of the lense for visible light
(essential when You measure the focal length), deltaN - the difference between
the refraction index for the wavelength of Your laser and the refraction
index for the visible light.
For example K8 glass (common optical glass, used for most of the affordable
lenses) the amendment between visible (500 nm) and near infrared (Nd laser,
1064 nm) is: deltaN/(N-1) = 2.1%. I.e. for a lense with FL=50 mm in visible,
the amendment will be +1 mm for the invisible light. For a lense with FL=100mm
the amendment will be +2 mm. These are rather low values, You may neglect in
most cases. More sufficient are the amendments between visible and infrared from
a CO2 laser (12% for ZnSe and 7% for KCl) or between visible and ultraviolet.
NOTES ON THE CRYSTAL GROWTH ←
For the russian speaking audience I may offer a reference to an excellent
wiki-resource devoted to the crystal growth: https://ru.crystalls.info/.
For the english speaking audience I don't know such a reference.
There are also many teaching aids from ones for the beginners to the
most advanced ones.
A very simple one may be found at https://www.wikihow.com/Grow-Alum-Crystals where a kind of classical school method using a jar and a thread is described.
I should add from myself that the "classic school jar-thread" method did not
gave any acceptable results. A simple for the homemade reproducing and use
Belustin's crystallizer did also behave just like classic jar with thread.
(I.e. it did not show any advanced capabilities.) A creation of some
complicated crystallizer with circulating thermostat and washer was out
of my resources, so I reduced to the method of "mining in the artificial
deposit."
The method is as follows:
- Determine the volume of the solution, which You will use in one round, and
find a proper vessel-crystallizer. The more solution You use the larger
crystals You will obtain, so it is senseless to use less than 1.5 liters.
Lets suppose that You have determined with the volume, and lets designate it as V.
It is desirable that the volume of the vessel be 20%..30% larger than V.
Good results can be obtained with plastic bottles of 2, 3 and 5 liters,
typically used for drinkable water. One can easily use ones from fresh water or
sweet drinks. Avoid usage bottles from mineral waters, they require too much
of distilled water to be washed properly .
- Measure the temperature at home, or in those premises, where You intend
to install Your jar with the solution (it can hardly be named "crystallizer").
You need to measure the temperature with the precision to 1..2 degrees of
Celsium. Not worse. When You've determined the room temperature, determine
the temperature of the solution's saturation. Let's designate it as To. It
should be 5..7 degrees higher than the measured room temperature. (For example
in my case the room temperature was 26 degrees, and the temperature of 32
degrees was taken as the design temperature of saturation.)
- For the chosen volume of water V calculate the amount of salt m, necessary
to make the solution saturated at temperature To. You should know the
solubility of Your chosen substance. For ADP and KDP i have a (homemade)
formulas:
ADP: m(ADP)=0.01179*To^2+0.28339*To+24.33 grams for 100 ml of taken water
KDP: m(KDP)=0.00545*To^2+0.26067*To+15.28 grams for 100 ml of taken water
To in these formulas is the temperature in Celsium degrees. For other
substances You should find the data on the solubility all by Yourselves.
CALCULATION EXAMPLE: one has a five liter jar, and wants to pour there about
3 liters of the solution. It is desirable that the solution be saturated at
31oC. The substance is KDP.
Using the formula for KDP:
m(KDP)=0.00545*31^2+0.26067*31+15.28=28.6 grams for 100 ml of taken water.
Scaling to 3 liters: 30*28.6 gram = 858 grams of monokalium phosphate.
- Measure the chosen amount of (distilled) water and weight the calculated
amount of salt. In order to have reproducible parameters the precision should
be not worse than +-1 gram. Not every kitchen scales have such a precision and
dont try to measure 3 liters of water by 100 ml rated measuring glass. Choose
proper measurement aids. A digital kitchen scales with the precision to 1 gram
at weighting mass of 4..5 kg can be bought in a supermarket if one has a bit of
persistence. I recommend You to weight the water too instead of measuring its
volume. If You weighted the water using the same scales, You've used for the salt,
the errors of weighting might be partially compensated. It is desirable to
weight the water directly in the vessel, where You will cook the solution.
Yes, You will still need another vessel, aside of the jar for crystallization.
It could be a large pan. Ideally - made of heat resistant glass. It is
rare and expensive however, so one can use enamelware if not too lazy to clear
it thoroughfully. A good compromise is stainless steel pan.
When You've poured the calculated amount of water into the pan, heat the water
to ~50 degrees of Celsium and put there the weighted amount of salt. Stir until
it dissolves. It is not bad to boil the solution for ~ 15 minutes. (Don't
forget to close the lid of the pan, otherwise the water evaporates and the
saturation point of the solution becomes unknown.) The boiling helps especially
if the solution refuses to settle the crystals of the desired shape (due to
the polymorphism). For example the solutions of KDP and ADP use to "get habit"
to the needle shape of crystals even if the acidity of the solution has already
been corrected.

NOTE: the method requires precise weighting. One might use another approach:
at the beginning one needs to prepare a solution saturated at the room
temperature, and then, with heating, to add there an excess of salt. The excess
is to be calculated for the proper difference between the room temperature and
the desired temperature of saturation. The calculation is simple: one needs
to calculate the amount of salt making the solution saturated at the desired
temperature of saturation and then subtract the amount that makes the solution
saturated at room temperature. For example if the room temperature is
25 degrees and the desired saturation temperature is 31 degree, the necessary
excess will be:
ADP: delta_m(ADP)=0.01179*(31^2-25^2)+0.28339*(31-25)=5.66 grams per 100 ml of water
KDP: delta_m(KDP)=0.00545*(31^2-25^2)+0.26067*(31-25)=3.39 grams per 100 ml of water
The absolute precision here is the same as above, but the relative error can be
significantly higher. It is simplier to weight 100 grams with the precision to
1 gram is much simplier than to weight a kilogram with the same precision.
The method has its own drawback however: it is a very slow one. If You think
that the solution is already saturated, when You are still stirring and the
salt does not dissolve, You are completely wrong. The real saturation can
be achieved only after the solution have stood three days at least over the
solids. A week is even better.
- When the necessary amount of salt has dissolved in the taken amount of water,
filter the solution through coffee filters into Your jar for crystallization.
During the filtration the temperature of the solution must be kept higher than
the chosen saturation temperature. Otherwise the crystallization may occure on
the filter and it will ruin the whole process. For this reason don't use too
fine filters (like chemical "blue ribbon" type) - the filtering in this case
may be too slow and the solution may cool down during the filtering.
- Close the crystallization jar by some lid and install to the place, where
you have measured the temperature (at point 2 of this process). If You can
equip the jar with a thermometer, You can insert a seed crystal after the
solution have cooled down to the saturation temperature (see poins 2 and 3).
However doing this is of little sense. To the end of the experiment the seed
will grow into a large crystal of very nasty quality.


- Wait for 2 days at least. After this time have passed the bottom of the jar
and its walls will be covered with a layer of crystals of different sizes. If
their size is too small for You, You can move the jar into more cool place
and wait further.
If 7 days have passed already and the solution still refuses to give
crystals, it may meant that You've choosen too low difference between Your
room temperature and Your desired saturation temperature (point 2). Or either
there was a mistake during weigting water or salt. It also may be that the
room temperature has suddenly risen and the jar needs to be moved into some
colder place.
On the contrary if the crystals settle too actively and You are not satisfied
with their clarity or shape, You need to reduce the difference between the room
temperature and the design temperature of saturation.
- If the result does not satisfy Your needs, correct the themperature gap
between the room themperature and the design temperature of saturation
accordingly to the notes made at point 7. Repeat the experiment. At the third
to fifth repeat You will start to get crystals of decent clarity with
the sizes suitable for the tests of laser frequency doubling.
- Pour the solution out (to some reserve vessel) and dissect the jar.
(Plastic jars are easy to be cut by a knife.) Scavenge the scrapyard of
crystals on the bottom of the jar in search of a suitable crystal. Essentially
what You've done is the artificial deposit of crystals and You are only
to find a gem of Your dream there.


NOTE 1: If during the gowth You notice that You've grown a crystal of
acceptable size and clarity, don't wait anything, stop the process immediately
and get the crystal out of there. It is because of high probability that the
crystal will become turbid or covered by "children" during the further growth.
On the other hand if You are looking into the jar and don't see anything good,
there is also a high probability that with further growth one of "those little
junks" will become a good one.
NOTE 2: If one takes a crystal out of the solution and then returns it back,
the crystal continues to grow but inside it a turbid surface of separation
appears. The optical quality of the crystal is then ruined. It happens even
if the crystal was out of the solution for only 1..2 seconds. The crystal
does not need to go completely dry for it to happen, so be careful and dont
disturb the growth more than it necessary. On the other hand, while the
crystals are completely covered with the liquid (and while they sit tightly
and dont peel off), You may shake or kick the jar as You like - the optical
quality won't suffer.
NOTE 3: For the growth of large and clear crystals the purity of the source
material and stability of the temperature are the factors of most importance.
If You are allowed to a chemical shop, I recommend You to get a stock of source
substances of the highest possible purity and not deal with fertilizers.
At home it is better to grow crystals in winter, when the room heating is on.
At this time the room temperature is more stable than in summer, when the
wind does what it likes. A good wine cellar can be a good aid too.
NOTE 4: If You decided to deal with fertilizers, You can try to combine
the described above process with a chemical purifying method, known as
"recrystallization". So at each circle You will get more and more clean
product. A commercial fertilizer grade monokalium phosphate (MKP) is usually
pure enough to get small (10..15 mm) crystals of acceptable clarity without
any additional prification. However an ADP containing fertilizers are usually
heavily contaminated by gypsum. They require thoroughful cleaning and
multiple times of recrystallization until one is able to get a first
transparent crystal. The situation is aggravated by the fact that the
turbidness continue to appear eben after multiple filtrations and
crystallizations. (The soluble calcium hydrophosphate hydrolizes and
precipitates in the form of unsoluble phosphate.)


NOTE 5: It is known in the technology of crystals that the higher is
temperature, the faster is crystal growth. However my own observations show,
that the clarity of the crystals goes better when the temperature goes lower.
The best crystals I got ("ice clear" ones) were obtained at the temperatures
between 5oC and 10 oC. So it may have sense to perform the growth in a fridge.
This behaviour may be due to contaminations - my experiments were with
fertilizer grade substances, not with analytical grade ones.
NOTE 6: If You do use seeding, You need to "activate" the seed before putting
it into the soulution. If a crystal was stored in open air for a while, it
won't grow once been put into the solution. (It can become covered with other
crystals, but it does not grow itself.) The "activation" procedure may look
like 30-sec bathing in a distilled water. Small seeding crystals can just
dissolve during this bathing. A happy idea - to bath the seeds by a saturated
solution yields nothin - the crystals do not become activated.
TESTS WITH LASER ←
The laser had a passive q-switch and a YAG:Nd rod 3 mm in diameter. Energy
of pulse was 2 mJ, the duration was 20 ns. (Both walues weren't measured.
They are calculated from the stimulated emission cross section of the Nd in
this crystal, from the initial transparency of the q-switch and from the
reflectances of mirrors.) Its beam had divergency of 3 mrad. It means the
evil multimode state. The polarization state at the output of the laser was
not clearly known too. (The intereference film polarizers for 1064 nm are absent on eb@y as class, and prisms of Glan or Nickol are
so expensive, that it can make Your desire to work with them to vanish.)
There are no polarizing elements in the cavity of the resonator, so it could
seem that the output radiation should be unpolarized. However, as I've
mentioned many times in other guides, lasers always tend to emit the light of
some certain polarization. The experience shows, that YAG:Nd ones without any
polarizing elements have the degree of polarizaton 60..80% commonly.

Here we come from a beautiful idealized theory to a severe practice. Having
100 kW in the beam of poor quality with the polarization unknown. Let's see
whether one can get some second harmonic using this thing at all.
Even the first tests have shown, that the powefull background light from the
lamp prevents one from seeing anything. The laser was then put into opaque
casing (see the photo above) and its beam was filtered, To filter out the
visible light one can use a HWB850 glass.
After these modification the second harmonic became visible.


Further on, to avoid the aligning of the crystal by finger, a small turnable
table was designed. Here are its photo and model:




It has only one axis of rotation, but as we can see from the theory (fig. 1)
it is enough. After tuning the angle the results became much better:


Green light from this laser was occasionally able to lase an organic dye
solution. However it was weak, rare and unstable. I was unable to catch any
photo. Sorry about this. Obviously such a result didn't suit anybody. Including
me of course. So a new laser of higher power was build specially for this very
guide. At first it used a YAG:Nd crystal with 4 mm diameter and 80 mm length.
Russian krypton lamp DNP-6/60 was used for pumping. (Using of krypton in place
of xenon boosts efficiency of Nd lasers making them more powerful.) The lamp
and the rod are placed inside elliptic aluminum reflector. Resonator mirrors
are commercial ones (one with reflection of 99%, the other with 50%). Q-switch
was also bought. It is a spare part of laser epilator and has a shape of
rectangular brick 8x8x4 mm. Its initial transparency is 20..25%. Such
Q-switches are available now at affordable prices
there.
Here i give the hyperlink to the shop, not to the certain lot, because lots
are short-living and the lot reference may become waste even during updating of
this page.
Storage bank of the laser: two aluminum electrolytic capacitors (manufactured
by Rec) rated to 470 mcf x 450 volts. Both capacitors are connected in series.
The charging voltage is rather low (600..800 Volts), and it means that the lamp
can not be flashed by external ignition electrode. So a series ignition circuit
was elaborated.

The switch on the scheme is either a pushbutton or a circuit of
synchronization with a photo camera. Note that most modern photo cameras allow
not more than +5 volts on their synchronization terminals. It means that in
place of the pushbutton on the circuit either very old cameras can work (those,
having been manufactured a long before digital era), or one needs to create
some interface board.
Some troubles have arose with designing of the ignition transformer
(L1:L2 on the scheme). About ten transformers of different design were tested.
(One was wound on 2000NN ferrite rings, another was on a rod from a radio-set
magnetic antenna, yet another was on E-shaped transformer iron core, etc.)
It was found that the secondary winding needs to contain about 100..150 turns
for stable ignition of shorter lamps (DNP-6/60) and about 200..250 turns for
stable ignition of longer lamps (DNP-6/90). The number of turns is almost
independent to core material. The primary winding consists of 2..3 turns of
some thick wire. The thickness of the wire for the secondary winding is
questionable. If one took too thin wire, the resistance of the secondary
becomes too large and the energy from storage capacitors dissipates in it
rather than in the lamp. It means poor working or not working laser. On the
other side if one took too thick wire, the size of the winding grows and
its inductance grows essentially with the size. Large inductance means long
pulse and even the saturation of the ferrite core is out of help here.
If the pulse becomes much longer than the upper laser level lifetime, it
leads again to poor working or not working laser.
Comparatively good tradeout was found when 200 turns of enameled copper wire
with diameter 0.8 mm were wound in three layers over a ferrite rod from
magnetic antenna. Inter layer insulation was made by mylar sheet. The
resistance of the secondary becomes 0.6 Ohm, which is still larger than the
resistance of the lamp in ignited state (0.3..0.5 Ohms). It is obvious that
more than a half of energy from the storage bank goes to heat on the resistance
of the secondary winding, so all the gain in laser efficiency due to the usage
of krypton lamp goes to waste. If the efficiency is of no importance for You,
one can say, that the laser just works. Its output and "special effects" are
very similar to ones from the very known SSY-1 laser head. Just as SSY-1 it
can ignite a laser spark in focal spot of lenses with short focal length. Just
as SSY-1 it cannot ignite a spark with long FL lenses.


Since this laser was not able to outperform SSY-1, and we already know what
gives SSY-1 it was decided to develop the laser
slightly further. Now it is based upon a YAG:Nd rod with 7 mm diameter and
110 mm length and a longer lamp DNP-6/90. The lamp and the rod are still inside
aluminum elliptic reflector. 3D-model for 3D-printing of the reflector may be
downloaded from there.
The storage bank is three stages in series. Each stage consists of a
470 mcf x 450 V (Rec) capacitor and 220 mcf x 450 V capacitor connected in
parallel. It means the full capacity of the storage bank is 230 mcf. The
charging voltage is 1100 Volts.
Attempts to use the laser with series ignition transformer (described
above) were not successful. There was a free run lasing, but it was unable
to saturate the Q-switch. Finally it was decided to refuse from the series
ignition to the good of external ignition (sacrificing the lifetime of lamp
in favor of usage convenience). The new pumping circuit is shown on the figure
below.

Despite the fact that number of turns of (L1:L2) transformer haven't changed,
the secondary winding now can be wound by thin wire (like 0.1 mm) which makes
the transformer to be small. However there appears a choke in series with the
lamp. The choke coil contains 50 turns of thick PVC insulated wire. Its
inductance is 40 mcH (if anyone is interested in). After these modifications
the laser became able to saturate the Q-switch and became able to ignite a
spark in focus of lense with FL=50 mm:


Considering the transparency of the output coupler (~50%) and of Q-switch
(~25%) one may estimate that up to the moment of start of lasing the rod
holds ~100 mJ of energy. If a half of this is emitted (the other half dies
on the Q-switch absorption), one can estimate the output of the laser by
the value of ~50 mJ. The pulse duration can be estimated as 10 ns. It means
that the laser gives about 5 MW of power. Nearly 50 times more than miniature
laser did (the shots of it are at the beginning of the guide). How much of
green will the new laser do?
Home-grown KDP crystal was installed in focal spot of a lens with FL=270 mm.
At the beginning it did not give much. Blessing in disguise it allowed to
make good set of photos of tuning. The snapshots below contain a series of
green spots during turning the crystal:






All times The crystal was turned towards the same side. As before it was
installed on a rotating plate. And the turn from the most left photo in series
to the most right one corresponds to slightly less than a half of turn of
the worm-screw.
Further on a polarizer was added to the resonator of the laser. The polarizer
consists of two thin glass plates (microscope coverslips) installed at Brewster
angle. So the scheme of laser became as following:

The direction of the Brewster angle was set to yield optimal conditions for
II-nd type synchronism (i.e. Brewster angle is rotated to 45 degrees in relation
to the basement of the laser) see fig 4.
And it went as smoothly as if buttered:


The dye has also lased. In all range of concentrations.






All these colors are from the same Rhodamine 6G. One can behold the
concentration tuning. One can also see that (as it was with large nitrogen
laser) three quarters of the green beam misses the cuvette, and it still lases
brightly.
The homegrown crystal in the focus of 270-mm lense is subjected to breakdown
from time to time, but it is usually able to endure up to a hundred of pulses.


A pair of words on the biaxial crystals at the conclusion. Yes it happens that
some crystals have not one optical axis but two of them. (No-no, the nature
LACKS tri-axial crystals, You know. It is due to the fact that our space is
three-dimensional. Would it be four dimensional there'd be tri-axial crystals
too.)
In the biaxial crystals the frequency doubling process cannot be characterized
by a sole angle of incidence relative to an optical axis. The allowed direction
(the direction of the beam that causes phasematch) is determined by two angles
here, and the surface formed by allowed directions is now not a simple cone,
as it was in uniaxial crystals (see fig 1), but a tricky 3D shape.
There is no ordinary ray in biaxial crystals. That's why one can not designate
phasematch type by "o" and "e" letters as it was before.
Since there are two optical axes in biaxial crystals, direction any ray there
may be described by two angles. But they usually calculate angles not between
the ray and each of the axes, but between the ray and both of the principal
planes. There are some conventions about the directions of the angles too.
They usually use literal designations "θ" and "φ" for these angles. If
the phasematch is possible in a particular crystal, it means that there are
infinitive number of the allowed "θ-φ" pairs. Generally these pairs form
a smashed (and sometimes even broken) cone of the directions, that was
mentioned in the paragraph above.
Among all possible phasematch directions there exist a certain distinguished
type, beloved by commercial crystal manufacturers. This is a so called "non
critical" or "uncritical" phasematch. It is when θ=90°, and φ is equal
to something else. For example, for neodymium laser frequency doubling in
KTP crystal θ=90°, φ=23.3°; and in LBO: θ=90°, φ=11.6°. Don't
let the term "non critical" to fool You. While the angular tolerance is indeed
somewhat less strict here, all the other tolerances are still as strict as in
the previous case. Those particularly are: the temperature tolerance, the
wavelength tolerance and so on. One can say that the uncritical phasematch
as does not like the poor beam quality, as the critical does.
Good commercial biaxial crystals have much higher effective nonlinearity, than
their uniaxial relatives do. They allow more effective frequency doubling at
lower level of power. However, biaxial crystals of the most interest can only
be grown from the melt. KTP and LBO are among them, and it lowers the interest
of DIYer to them strongly.
- M.P. Shaskolskaya. Notes on Crystalls Properties. M.: Nauka, 1978
- W. L. Bond. Crystal technology. Stanford university, 1976.
- Russian textbook on optics: Butikov E. I. Optics. M.: High school, 1986.
- Russian textbook on crystallography: M.P. Shaskolskaya. Crystallography. M.: High school, 1984.
- SNLO nonlinear optics code available from A.V. Smith, AS-Photonics, Albuquerque, NM.
http://www.as-photonics.com/snlo
<< HOME PAGE |



