. : Powerfull Air Nitrogen Laser (Guide) : .

Following this guide You will learn how to cook a powerfull nitrogen laser, working on athmospheric air as an active medium. The term "powerfull" deserves a few comments. For a person having been related to nitrogen lasers for some time even the single millijoule is perceived as damned huge amount of output. And also the issue of the correct measurements of nitrogen laser output energy is also dark and cloudy. This is my own point of view, but I dont trust in more than 50% of published results related to this topic (look further in the "commentaries" division).

In the process of determining what lasers deserves to be called as "powerfull", one can use the opposite approach - to look from the point of view of the application. It is well known that most of nitrogen lasers are used to pump dye lasers. The Internet contains huge amount of homemade designs of nitrogen lasers. However only a few of them can drive a dye laser to the threshold. Even fewer ones can do it by the direct (unfocused) beam.

In this guide we'll use the term "powerfull nitrogen laser" in the meaning that it can drive a cuvette with a proper dye to the lasing threshold by the direct beam. Its obvious that it won't cut wooden boards or ignite a spark in the air.

1. RESOURCES

We will need:

1. Something flat and hard for the basement of the laser. (The nitrogen laser teaches to see the other side of the things. The items, that You earlier considered to be flat, appear to be too curved. Things, that You earlier considered to be rigid, begin to show unacceptable bend.)
   In my particular case a rectangular sheet of plexiglass (10 mm thick, 300 mm wide and 400 mm long) was taken as the basement. It's a pity but such resources aren't freely lying on Your road. The thing was bought in a special shop by a special order.
2. Mylar sheet 0.12 mm thick and having enough area to cover the entire basement of the laser. Even better it is to have some surplus. The ordinary polyethylene cannot serve as a dielectric for powerfull nitrogen laser. It has poor electric strength and poor heat strength. The last will come to use when the laser begins to emit heat in places of imperfect connection or where the corona discharge glows. Mylar can be replaced by kapton. However it is much more expensive and the output will be (according to my experience) generally at the same level.
   After some moment a whine, like "I am a simple student, where me to get Your fancy mylar?" is not accepted. Any supermarket sells baking sleeves (aka "oven sleeves", "oven bags", "cooking sleeves", "frying sleeves", etc, etc...)
   
   The thickness of the mylar foil in the baking sleeve is of course less than needed. But one can put several layers. Measure the thickness of the foil available to You and put as many layers as needed to obtain the total 120 mcm. Usually it will be 6 layers or (if we remember that the sleeve consists of two layers of film) it will be 3 double layers.
3. Kitchen aluminium foil.
   Get the most thick among all available. It is rather easy to find one up to 14 mcm thick. It is thinner than desirable, but further i will explain the workaround.
4. High voltage power supply rated to 16+ kilovolt.
   The description of making one almost without applying might and mind is placed here.
   It also appears that he laser may be fed by a common stun gun. The one should have its sparklength over 16 mm. If You have les powerfull stun gun, You may use a pair of them in series. How to do that is described here.
5. Some planar steel sheets. Steel scholar rulers are good here. Since the steel is needed to perform a magnetic attachment, You should check Your rulers whether they are magnetic.
6. Six pcs of small magnets. (The stronger the better.) Ideally use those fancy neodymium magnets.
7. Double gapped spark gap of rail type. (Sometimes they call it rail switch.) For not to make the description twice, in this guide it wont be described. Refer here for the procedure of its creation and tuning. The rail spark gap has sufficiently lower inductance and switching time. It improves the power of the nitrogen laser reasonably well. If You are too lazy to make the rail switch, I recommend You to refuse from the winged design in favour of the charge transfer one (see here in the"commentaries" part)
8. The material for the electrodes. For the sake of simplicity I will write that You will need a pair of aluminium strips 2.5 to 3 mm thick 2 to 3 cm wide and 30 cm long. Further on in this guide You will find that many other resources will suit. Full range for Your fantasy. The main requirements are straightness and the sizes allowing to make the necessary shape.
9. A cuvette with a laser dye. The cuvette will be used not only in order to test the laser's operationability, naturally it is the very instrument for tuning the laser for maximum power. The cuvette and the dye may be commercial. On the other hand the cuvette may be homemade (how to make one You may find here) and the dye solution may be replaced by a juice of one of lasing markers (the list of markers that have been tested for lasing You may find here). Finally one may try to tune the laser for the maximum power using a photodiode/photoelement as a sensor. However it is less spectacular and requires more skills.

Some other minor resources required:

10. stranded wire
11. solder and flux
12. alligator clips
13. in some cases there may arise a need for a ballast resistor rated to 1..10 MOhm. (The main requirement is that the resistor should endure the power supply voltage without the flashovers)

Tools:

1. scissors
2. hacksaw
3. drill (with bits and felt disc)
4. rough sandpaper (#200..300)
5. fine sandpaper (#1000)
6. polishing paste capable to work with metal (it may be automotive polishing compound)
   
7. Filers (fine and rough)
8. a caliper (dont expect that a common ruler will suit. You will need to measure the gaps with 0.1 mm precision)
9. screwdriver with a very big dielectric handle. (driving the screws will be the last thing You will do with this tool. Mostly it will be usefull for the alignment of the electrodes and for discharging the laser after its turn-off).

2. PRODUCTION OF THE ELECTRODES

In our design the electrodes should have 300 mm length. (You may choose longer electrodes, in this case be sure to elongate all other parts of the laser proportionally).
The working edge of the electrodes has a shape of a knife with steep angle sharpening.

The picture shows the cross-section of the electrode near the working edge. The dashed line shows the adjacent electrode.

The statement that the air-filled air-pressure nitrogen laser should have sharp shaped electrodes for the best performance was found out by at least three human beings: Alfonso Rodriguez, Jarrod Kinsey and the author of the present guide. They did it independently and were guided by different ideas but the conclusion, they came to, was the same.

The angle of electrodes sharpening is determined by two things: the thickness of the electrodes (the height of the point, where the working edge is placed, above the dielectric) and the fact that the gap for the sliding discharge H should be nearly twice as wide as the gap of the main laser channel h. In its own turn the gap in the main laser channel should have nearly a half of the value of the gap of the main discharger (rail switch) that drives the laser to action (for the explanation of this fact refer to the commentaries section). Since we use double gapped discharger with the nominal gap from 2x2 mm to 2x3 mm, it means that the main laser channel width may vary from 2 to 3 mm.

The distance between the electrodes at bottom (along the dielectric surface) should be choosen twice as wide as the main laser channel H=2h. This relation is flexible to a certain extent. Just remember that if You choose too small H, Your laser will be shortened by the sliding discharge along the surface of the mylar foil. On the other hand if You choose H too large, the sliding discharge will be too weak to preionize the laser channel effectively - it will be too hard to obtain a uniform glow between the electrodes. If we divide this necessary incrementation of the distance between both of the electrodes, we will get the necessary size of the chamfering: delta=(H-h)/2=h/2=1..1.5 mm.

The height of the working edge placement above the dielectric (the thickness of the electrodes) is rather flexible value too. However if it is too small (~1 mm and less) the discharge will have a tendency to slide to the dielectric, thus preventing the normal laser operation. In case of too large height (~5 mm and above) the laser channel appears to be too far from the preionizing discharge, the preionization efficiency decreases and the power of laser drops (in the best case... in the worst case You get inoperational laser). Generally one should better try to place the working edges of the electrodes at minimal height, where the discharge is still stable. Practically one may choose the thickness of the electrodes from 2 mm to 3 mm.

Naturally thats all the considerations that determine the sizes and shape of the electrodes near their working edge. The remaining part of the electrodes may have arbitrary size and shape with keeping in mind the good abutment to the wings (wings=metal foil plates) and good mechanical stability (stiffness). Its obvious that the electrodes alongside should be as straight as possible. (The light goes along the straight line and there are no affordable means to bend it in order to follow some curved electrodes.)

I was able to find the admissible parts in a builders store in a form of aluminium constructable doorhandles. Their main part has a shape of prism with a section that resembles a right angular triangle. If we put this prism onto its cathetus face, its ornate chamfers will form the necessary acclivity under the sharp edge.

So suitable resource did really reduce the number of processing operations. But I'm pretty sure that in place You live in, those doorhandles are unavailable. However there are many other suitable things, just go shopping keeping a keen eye onto the shelves. If nothing suitable is obtainable one could take a simple aluminium strip, 2..3 mm thick and 20..30 mm wide and make a necessary bevel. I.e. transform the strip into a blade with a steep angle of sharpening. Machining here is the best, but naturally one can do it manually using a filer. On the picture below is the sample of such an electrode made of aluminium strip.

If the working edge does not want to become sharp - dont worry. Too sharp edge is too weak to the affect of sparks. And it is almost impossible to avoid any sparks at least during the tuning procedure. To be a bit stronger the working edge must have some dulling. Its roundness should be barely seen by eye, like a dull knife has.

All other sharp shapes (except the working edge) should be rounded by a filer. It will allow to avoid much of corona during the laser operation.

If the blank is anodized - remove the anodizing by a rough sandpaper.

The working edge should be polished. One can do it using a polishing paste, drill and felt disc. Naturally the procedure is not as hard as it seems.

If the working edge for some reason has a curvature or scuffing, it should be leveled and grinded (sorry my tongue refuses want to say "ground", since the ground sounds like earth surface to me). Use a sheet of #1000 sandpaper, glue it to a long straight and hard part (a sheet of window glass or a piece of aluminium angle extrusion and so on) and grind with leveled motions with even pressing. After the working edge became straight and flat - just polish it.

The correct electrodes have straight flat shiny working edge with some dulling (dull knife). If one puts them with their working edges connecting one to another, and look at some light through them, the maximal clearance should be not more than a human hair thickness.

NOTICE: though the laser is operational even without the polishing (with grinded electrodes) dont disregard the polishing stage - it gives a serious gain in the laser yield (2..4 times).

3. ASSEMBLAGE

The rail spark gap may be attached by bolts or by screw clamps. If You plan to use bolts, then You first operation will be to drill the holes for the attachment bolts. Place the discharger to its further position, mark the suitable positions for the holes and drill them.

Take some sheet of steel foil, or steel rulers and glue it to the area, where the electrodes will be placed. It will be the baseplate for the magnetic attachment of the electrodes. At this stage use some elastic glue (like rubber one). Yes one can use weights or plastic bolts to press the electrodes, but the magnets are more robust and they work independently of the gravity force. Ideally the laser may appear to become portable.

The area covered with steel should cover the whole place of the possible motion of the electrodes. In our case it is 30x6 cm near the axis of the laser. It bugs to stumble over the steps under the dielectric. So if You want to avoid this, it is better to cover all the working area of the baseplate with steel (as it is shown on the photo). If Your baseplate is already made of steel, surely You may not bother to add additional covering.

Cut the grounding plate (common conductor) from the aluminium foil. Place it onto the baseplate and flatten. One may fix it with small pieces of a thin sticky tape. Otherwise one can leave it as is - it will be pressed by the discharger, by the electrodes and even by the electrostatic forces, so it will unlikely be able to move anywhere.

Above the grounding plate put the mylar sheet. Flatten it. The most thoroughfull flattening is needed if You use a multilayer film (cooking bags etc.)

Further on one needs to make wings. It's best if You can use rather thick aluminium leafs. However most of us will use thin (14 mcm) aluminium foil due to the reasons of availability. Thin foil tends to concentrate field around its sharp ends. This leads to corona and in turn to dielectric damage and flashovers. To reduce this harm one should make the wings from several layers of the foil. The folding method is shown on the photos below.

The reason of such a twisted folding is to avoid the sharp foil edges at the perimeter of wings and to place there the folds with noticeable rounding radii. If You can provide this by other method of folding - just fold as You like.

The size of the wings is 30 cm x 10 cm. The length is equal to the length of the electrodes and the width is equal to 10 cm. Decreasing the width of the wings below 10 cm gives a severe penalty in laser yield. Almost proportional to the reduced area. Increasing the width over 10 cm causes slow rise of the output power. In addition it causes increasement of the stray sparking. Above 15 cm it is really hard to say whether there would be any increase of power if there were no sparking.

Having put the wings down one should check the capacitance between each of the wings and the grounding (common) plate. One could use a multimeter able to capacity measurements. Dependently to the quality of the
assemblage You should get from 3 nF to 5 nF (per each wing). If the capacity is too large You probably use too thin dielectric (it should be 0.12..0.15 mm thick) If the capacity is too low - it most probably signs that there is too much of inflatness and bubbles there.

Here is the photo of the wings having been put inplace.

Before putting the electrodes it has sense to make some contact spring stiffners. They are intended to provide good contact between wings and electrodes along all the length of the electrodes. In practice the electrodes will never abut to the baseplate since they are straight and the baseplate is not perfect. Irregularities and bubbleses will make things even worse. And the contact in the nitrogen laser must be not "just good" but "perfect". When the laser fires there flow kiloamperes of current and every excessive milliohm affects the laser yield.

The spring contact stiffners may be made using the same aluminium foil, folded several times and corrugated. The length of the stiffners should be equal to the length of the electrodes and the width should be 10..20 mm

Put the stiffners over the internal edges of the wings (along the edge, where the electrodes will sit). Above that install the electrodes. Press them down. The pressure force should be enough to shear the stiffners.

Fix the electrodes by magnets.

Install the rail spark gap. In places where its leads lie onto the foil its also desirable to use stiffners in order to enhance contact.

If You use multilayer mylar, it has sense to put some plastic (dielectric) plate (2..3 mm thick) just below the discharger between mylar layers. It may be a cut of school ruler or something else. This technique will reduce the flashovers under the discharger, where the presence of ground plate tends to stimulate them.

It remains only to make an electric contact between wings for the constant currents. A small coil of 3..4 turns of strand wire suits well. The leads of the coil may be soldered to alligator clamps and it helps to attach and detach the coil without ruining other things.

Low number of turns in the coil helps to suppress the stray sparking. However it isnt the panacea.

After installing the coil to its place the laser is ready.

4. TUNING

Tuning of the laser consists of two stages:

• tuning of the rail type spark gap (the rail switch)
• tuning of the laser itself

In order to tune the rail spark gap, take the fresh-assembled laser, set the laser electrodes to be parallel to each other and set 2..3 mm spacing between them. Then tune the rail spark gap. Its tuning procedure has already been described in the spark gaps guide . Its main sense is to obtain more or less even sparking along the spark gap by means of changing the pitch of the central electrode. If the spark shines only in a single place, Your rail spark gap has almost no advantages against a common pinpoint type one.

Usually one can get 2-3 sparks simultainiously in one (first) of the gaps and 3-5 sparks in the another (second) gap without too much of torture. This gives a serious reduction of the inductance and increasement of speed. By very thoroughfull tuning after many iterations one can get even better results. However its a bit excessive for this type of laser.

Having tuned the spark gap one can advance to the laser tuning stage.

For the correct tuning one should keep in mind the next three things:

1. Laser has a preferencive direction of light. If it was assembled accurately (with no bubbles under the dielectric, with evenly lying wings and with evenly pressed electrodes) the preferencive direction is towards the spark gap side. The reason for this phenomenon is due to the dissipation of the electric energy (i dont like the word 'wave' in this context) when it travels from the spark gap to the far away end of the laser electrodes. The amplitude of pulse drops and You are forced to make the spacing narrower there. And it immediately causes the laser to 'push' the light towards the side of wider spacing. i.e. towards the spark gap. The longer laser is, the more prominent this phenomenon becomes. Of course one can align the electrodes to have more narrow spacing near the spark gap and more wide at its far away end, thereby forcing the laser to emit in the opposite direction. However it leads to the situation, when the strong pulse is applied where the spacing is narrow end and the weak field pulse is applied where the spacing is wide. At the narrow place this causes sparking and arcing and at the wide place there's almost no discharge. It means less overall power than in case of the laser having been aligned properly.
2. If the laser electrodes are not parallel to each other, they form some wedge (see the picture below). The light does always prefer to go towards the side where the spacing is wider (size h2 at the picture) rather than towards the narrower side (size h1 at the picture). It is due to the fact that the time it takes the electric discharge to cover the wider spacing is always more than the covering time for the more narrow one. It causes the discharge to form a kind of "travelling wave". And of course if the light goes into the same direction as the travelling wave does, it experiences more amplification in the freshly pumped medium. In addition to this there exists a kind of trumped phenomenon. I.e. the light travelling in the diverging waveguide tends to be pushed out towards the bigger end. And the reflective metal electrodes do the job nicely. The wedge directs the light so strongly that it allows to force the laser to emit into the direction opposite to the preferenced one. The existence of the preferenced direction can hereby be noticed only if one compares the top achievable powers for the cases of aligning the electrodes for one direction and for the another.
3. There exists an optimal average value of the inter electrode spacing h=(h1+h2)/2, at which the output power is at maximum. As it was mentioned above, in the first approximation the optimal value of h is equal to the half of the value of the gap in the main (driving) spark gap. However it is only approximation. The precise value of the h is to be found in the process of tuning.
   

Thus the tuning of the laser is the process of searching for optimal spacing between the laser electrodes and the optimal angle between them. Here we have a two parametric optimization problem.

A single parametric optimization problem is simple. A two parametric optimization problem is disgusting. And the three parametric optimization problem is the one, u should avoid if possible.
So this disgusting problem have to be solved during the laser tuning.

Namely due to its disgustness, I doubt that anyone wants to solve it too often, so: dont forget to write down the resulting values of h1 and h2! In future this writing will come in handy many times. If You literally followed the guide and the important sizes of the laser (gaps in the spark gap, sizes of wings and electrodes) are just the same as here, the h1=2.5 mm and h2=2.7 mm would be a good initial approximation.

In order to set the spacings it is handy to use drill bits. Take two of them having 2.5 mm diameter. Wrap the shank of one of them with a sticky tape or thin paper until it diameter reaches 2.7 mm. Use a caliper to measure the resulting diameter. In other case You can use a 2.7 mm drill bit if it is available to You.

Insert the shank of the 2.5 mm drill bit between the laser electrodes somewhere closer to the end far from the spark gap. Insert the 2.7 mm bit between the electrodes somewhere near the end being closest to the spark gap. Press the electrodes one towards another until they clamp the drill bits. Then extract the drillbits carefully.

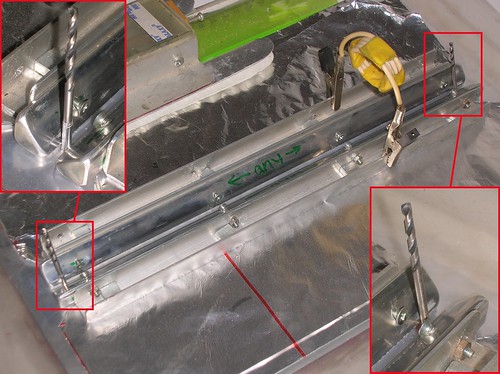
Having turned on the laser, most probably You immediately will observe a purple glow between the electrodes (maybe with some stray sparkies). There will also be a bright spot if a fluorescent paper was put on the way of the beam near one of the laser ends. If there is no glow between the electrodes it means that something has gone completely wrong and it is needed to open the laser and research for the reasons. If the glow is present but there's no lasing, first of all look for the beam at the other end of the laser. Then try to change the average spacing between the electrodes (usually towards reduction). If there's still no lasing, check the straightness of the working edges of the electrodes. Also check the quality of the electric connection between the electrodes and the wings. (However bad connection usually affects the glow - it can be seen in the form of dark or dimmed places).

After having obtained the lasing, start to tune the thing towards the top power. Now You will need a cuvette with laser dye. Take a commercial one or glue it Yourselves. Fill it with a laser dye solution to somewhere 2/3 of its volume. The solution concentration should be suitable for nitrogen laser pumping. One should take rhodamines or coumarines in 3..7 mmol/l concentration. Maybe it is not wise to use fluorescein here. It is capricious in power and concentration. If You have no experience with the dye lasers it may be more wise to get a certainly working solution. E.g. TextileWhite bleacher or a juice of yellow-green highlighter (a list of working ones You may find here and here). If You want to make the cuvette by Yourself, keep in mind that the glue seam shouldn't block the beam path of the dye lasing (see the figure below)

NOTICE: non lasant light sources normally do not emit the radiation with a prominent pattern (directivity). On the contrary in order to obtain a directed beam they use some concave mirror, lenses, apertures and so on. The simple fluorescence is not lasing, so its light will produce a more or less even exposure of the screen (sheet of paper) being placed aside near the cuvette. If You observe a stripe of light there's a high probability that it gives the evidence of lasing. (And the more sharp this stripe is the higher that probability.) If You observe a prominent spot - the conclusion is obvious.

Set the cuvette with the dye solution on the way of the nitrogen laser beam as close to the electrodes as possible. If the nitrogen laser was assembled correctly, the cuvette is good and the dye solution is suitable (see above) then You should see a light spot on a paper placed aside of the cuvette. If no, try to adjust the inter-electrode spacing. Dont shy to check the dielectric layer for the bubbles, check the electric connection between the wings and the electrodes, check the correctness of rail spark gap settings (the gaps in there should be not less than 2 mm, and there should be several sparks in parallel when it fires.)

Then arm Yourself with a screwdriver having a huge dielectric handle. Hit the electrodes gently to adjust the angle between them. As the output power grows up, increase the distance between the dye cuvette and the end of the laser. If You missed the optimal angle (and unless You got some experience You will miss it oftenly), interrupt the procedure, turn off the laser, discharge it, and then take the drill bits and renew the setting of the spacings. Then start to align the electrodes again.

SAFETY WARNING. If You are making a laser following this guide, You seem to be rather experienced person in lasers and high voltage stuff. It is kinda awkward to remind such a person that one may touch the electrodes only when the laser is switched off and all its capacities are discharged.

The laser, if having been correctly assembled and tuned, provides lasing of good dyes in rectangular cuvettes at a distance of 40..50 cm without any lenses or focusing. (The real laser grade high purity dyes have low threshold and can be driven at 100..120 cm.) With aged or burned electrodes this value can drop to 25..35 cm. If You cannot achieve 40 cm during tuning (and You are sure that You have a proper dye solution). I recommend check the electrodes and repolish the working edges of them if needed (especially since they could be fried seriously after some failures in the tuning process).

If for some reason You can not achieve lasing of dyes in the direct beam (maybe the proper dye was not available, or some other problem) You still can tune the laser using the cuvette with dye. Use a lense here (Better - cylindrical one.) As the cylindrical lens one can use some glass tubing filled with water or even a piece of round glass rod (in the last case one should check if the glass of the rod transmits enough light at the nitrogen laser wavelength. If You are using a lens, then the measure of the output power would be the maximal displacement of the cuvette from the lense's focus rather than the maximal distance from it to the laser's electrodes. Tune the laser using the lens until the maximal shift from the focus (when the dye's lasing is still present) becomes comparable with the focal length of the lens. Then You should be able to use the direct beam.

There's no limit to the perfection. And it is understandable that as Your laser becomes more powerfull, You want even more. However it is better to stop at some point. When You think that the results of tuning are good enough, carefully measure the spacing between the electrodes at the front and back ends of the laser. One can try to use a caliper, but it is better to use the drill bits again. Wrap their shanks with a thin tape until they fit closely the gap between the working edges of the electrodes. Then measure the obtained diameters and write them down.

5. OPERATION

Despite its simplicity the laser appears to be a serious device that will be of the very use for You further on. As to myself, it helped me to discover a plurality of laser dyes among common and readily available materials, it helped to choose the substance for a protective UV filter etc etc...

If You have a powerfull power supply, the laser should be operational at least up to 100 Hz (the limiting repetition rate for the uncontrolled air filled spark gap without forcefull blow). However I didn't check it at the repetition rates higher than 30 Hz. Because it's scary. First of all - the noise. The next is that the heating becomes very prominent at higher repetition rates. And the last is the lifetime.

Concerning the noise - I urgently recommend You to take the measures for its suppression. The most simple thing You can do - is to cover the laser channel with a dielectric plate. If You feel ready to design a more serious covering dont forget to cover it with rubber from inside (or with another sound absorbing material).

Concerning the lifetime - get used to keep a peaceful mind each time as the dielectric under the wings fails. Try to convince Yourself that the mylar is an expendable material. Just replace it, set the electrodes again, and continue the work as if nothing had happened. Remember that the laser works near the limit of the mylar's dielectric strength. In order to provide a practically eternal life to it, it would take to reduce the voltage more than by two times, and this will turn the laser into a useless toy.

Even SwissRocketman (known by his amazing videos on YouTube) reports a mean lifetime of the dielectric in his lasers at the order of 10000 pulses. And he is a professional, not amateur. By the way try to divide 10000 pulses by 30 Hz. It appears that the laser has a resource of only 5 minutes of flawless operation. And with 1 Hz repetition rate it gives about 3 hours.

Dont forget to discharge the laser's wings after You've finished Your work. Not only it will preserve You from a spontaneous electric shock, but also will prolong the time before the next replacement of the dielectric. When the mylar stays under the voltage it expends its lifetime in vain.

Aluminium electrodes have limited lifetime too. They tend to become fried. So every 2-nd or 3-rd replacement of the dielectric should be accompanied with the renewing of electrodes' working edges. Arm Yourself with a drill equipped with a felt disc and polish the working edges carefully. After several repolishing procedures it may be needed to flatten the working edges by some grinding tool. Alfonso Rodriguez reports that the maximal lifetime one can obtain with electrodes made of "inox". As far as I could know the inox is a kind of stainless steel. Moreover it seems to be a very specific kind. It is because the steels, especially stainless ones, are the compounds with a high specific resistance. And in accordance with this fact all my attempts to make a nitrogen laser with steel electrodes lead to fail. Its curious, but the discharge looks much better with steel electrodes. It is more uniform and has less sparks (or even free of them). But the laser output with the steel electrodes appears to be miserable. Judging of that the INOX appears to be able to register both of the useful properties: it is strong to corrosion and it has good conductivity. Other high conductive materials: bronze and copper will work not worse than aluminium does. However they also have similar corrosive strength, that means they will require cleaning as frequently as aluminium.

After the long storage the electrodes are also recommended to get some polishing. It will free You of the barren search of the places "where the power has gone to".

About the drawbacks

The laser has rather high spread of the output energy from pulse to pulse. The order of this spread is up to 50% (thanks goodness it works without omissions). First of all it is due to the instability of the firing voltage of the (uncontrolled) spark gap, and in the next turn it is due to the discharge instabilities in the laser channel filled by air (oxygen contaminated nitrogen). Using the triggered spark gap and filling the laser with pure nitrogen one can eliminate these instabilities, but the design complexity will be the price. On the other hand one may notice that even with such a high spread from pulse to pulse the average power (averaged over 10..100 pulses) is very stable.

The replacement of the mylar (with the related necessity to remove the electrodes) arises pain in the pass if the laser have been aligned to an optical scheme. However one should just think beforehand about this. Choose one of the electrodes to be a reference one (the one that sets the direction). Trhow an additional alignment ray along it. And after the reassemblage the reference electrode can readily be set along the alignment ray, and the other electrode can be easily aligned to the reference one. It should be mentioned that if You already have the sticks of the necessary diameters (e.g. drill bits that You've used on a stage of the alignment of the laser), You will only need to set the necessary spacing at the front end of the laser and at its back end. No additional alignment or adjustment will be needed.

By the way the laser is a powerfull electromagnetic pulse generator. I've never seen a cell phone killed by this small atmospheric nitrogen laser, but a few transport/credit cards with RFID chip it did fry. It usually happens like this: You forget the card in the breast pocket, then You come close to align the electrodes, and the next day it appears that the card is dead. So the expensive electronic devices should not be placed closer than several meters from the working laser. However it happens rather rarely. Some devices had a long life close to the nitrogen laser and still alive.

6. Comments

* The Shape of the Electrodes

The fact that sharp electrodes give more power may be described by the next three factors:

• field concentration
• increasement of the preionization
• decreasement of the operational volume with correspondent increasement of the gain.

Let's begin from the end of the list.

How can the value of the gain affect the value of the output power?
Two cases are possible here:

1. saturated gain, when the amplificated beam just gathers the energy stored in the volume of the gas
2. unsaturated gain, when the beam is weak enough to gather a sufficient part of the stored energy, so the amplification is limited to the gas gain ability.

In case of the saturated gain it's obvious that the output energy is proportional to the volume, it is being gathered from, multiplied by the energy density. And this product does not depend to the sharpness of the electrodes. Narrower edges - the volume decreases but the density increases. Wider edged - the density decreases but the volume increases. When the energy deposition per unit of length of the electrodes is given, its output energy is proportional to the length of the laser diminished by some small starting part.

In case of the unsaturated gain the output energy grows exponentially with the length of the laser. Each centimeter of the excited gas amplifies the light by several times.

However practically we don't observe thousand times growth of the output with every surplus ten centimeters of length. On the contrary the linear growth looks more trustworthy. I.e. we deal with a saturated gain and the concentration of the energy deposition can affect only the length of that 'starting' part - the length of that part of the laser, where the light becomes amplified to the intensity that it begins to gather the sufficient part of the stored energy. This length is rather short (3..7 cm) when compared to the total length of the laser.

Thus the third factor (increasement of gain due to increasement of the energy deposition density) goes away.

Concerning the second factor - it is the very fact that applying a preionization or either increasing its power in most cases affects lasers positively. Moreover the sharp edged shape of the electrodes was often used in the laser technique with the aim to preionize the discharge gap. The sharp electrodes were told to have different properties: from the ability to emit electrons due to autoemission to the ability to emit ultraviolet radiation due to corona discharge taking place in the process of voltage grow before the main discharge. It looks like the nitrogen laser with sharp edged electrodes has two sources of preionization: the sliding discharge above the dielectric between the main electrodes and the predischarge corona from their edges.

The relation between their intensiveness/effectiveness is easy to understand if we make (in mind) two experiments:

1. To create a laser with dull electrodes (e.g. made of tubes)... In the reality there's no need to perform such an experiment - it is clearly known that such lasers exist and perform well. They show good purple glow (volumetric discharge) and lasing. It means that they have enough preionization for glowing discharge and presense of lasing.
2. To assemble a laser with sharp electrodes and to test it in the absense of the sliding discharge. Actually there are 1001 way to suppress the sliding discharge or to make its preionization neglible. One may increase the distance between the dielectric and the working edges (i.e. increase the thickness of the electrodes), one may put a narrow paper strip onto the dielectric between the electrodes; one may create the electrodes of such a shape that the spacing between then will become too large (size H at the pic1) etc, etc. However it is unnecessary too, - at least in Alfonso Torres Rodrigues blog, devoted to nitrogen lasers there described a lot of such faults, including one of his very first tests, where the attempt to lubricate the dielectric with oil caused suppression of the preionization and absence of lasing. In such cases it appears to be impossible to get even the glow, not to mention the lasing. I.e. the power or the effectiveness of the sharp electrodes alone is not enough even for obtaining the volumetric discharge type.

Here goes the conclusion: the preionization due to the corona at the sharp edges is neglible when compared with the preionization due to the sliding discharge radiation.... Second factor gone away.

As to the third factor - the deficiency of the field tension in the discharge for the effective excitation of the nitrogen has already became a legend. Actually the electric field strength needed for the volumetric discharge ignition in the air is about 30 kV/cm (39 V/cm*torr), and they say it needs much more. E.g. Jon Singer after the review of the literature reports 86-100 V/cm*torr [see A Simple Nitrogen Laser Using “Doorknob” Capacitors in a Voltage-Doubling Circuit, and with Semiconductor Preionization, Primarily Intended for Do-it-Yourself Laser Hobbyists ] However there exists a suspicion that even this value was obtained merely as the ratio of the storage charging voltage to the laser channel spacing. However one may recall that the field strength in the real discharge has nothing to do with that. One may also remember that the field tension having place in the common self sustaining discharge is more suitable for effective excitation of vibrational levels in the far infrared CO2 laser, rather than for excitation of electron levels in the ultraviolet nitrogen laser. The energy height of the vibrational levels is ~30 times lower than the height of the electronic ones. One may come to a conclusion that thus the necessary energy of the electrons will be 30 times higher. The same will also be true for the E/p ratio. It means that the fields needes for the optimal excitation of the electron transition levels in the nitrogen laser would be as high as 1000 V/cm*torr. It is understandable that such a field is unreachable whatever gases You use or whatever efforts You put. It means that one can never jump across the optimum, and any increasement of the electric field strength will increase the effectiveness of the nitrogen laser.

It's to be mentioned here, that the electrical field by itself does not excite any atoms. Only the electrons are responsible for that. (You read "electric current") So it's of no importance whatever the voltage had been applied across the laser channel before the current has started to flow. It is important only how strong was the field inside the plasma during the active stage of discharge glow. And this actual electric field strength is extremely hard to affect. Any attempts to increase the applied voltage are parried by the gas by practically instant increasement of conductivity. Any excess of the feeding voltage are dissipated on the internal resistance of the power supply, not on the resistance of the plasma. And this plasma has much more reserves to increase its conductivity, than we have ones for the reduction of the internal resistance of the power supply (in our case - for the reduction of the discharge time of the laser wings). So it is the game with one gate. The only place where the speculations are possible is the real speed of this "practically instant" reaction of the plasma. However judging from the absence of the nitrogen lasers with really high efficiency it is able to drop the excess of the voltage fast enough for us to be unable to do anything with that.

Another way for the increasement of the actual field tension is to change the composition of the gas mixture. It is classic to use sulphur hexafluoride (SF6) for this purposes. The literature contains number of reports that the presence of SF6 in the gas mixture dramatically increases the efficiency of nitrogen lasers. Actually SF6 would also come handy for spark gaps filling - it would allow to have all the superiorities of pressurized spark gaps already at the ambient pressure. The problem is only that it is hard to find a tank of SF6 lying readily on Your road. And it is hard to replace it with something equivalent. There exist other gases with comparable electric strength (sulfur dioxide SO2 ~150 kV/cm, some freones ~200 kV/cm), but the freons tend to pollute everything with a soot appearing in the process of their decomposition in the plasma. Evidently it does not make laser tubes or spark gaps to work better. Sulfur dioxide SO2 is rather hard to produce and it stinks. However its main decomposition product is just the common sulfur, that is good dielectric and makes no harm. Possibly SO2 is the additive of the choice for DIYers.

For obvious reasons the pressure changes are not the subject of the discussion. First of all, we consider the atmospheric pressure laser. Second - since only the electron energy is important rather than the electric field strength, it comes that not the E itself, but only E/p ratio is meaningful. And at normal pressure range it does not vary.

However the things aren't as obvious with non-uniform field. First of all one should keep in mind that with concentrating the field in one part of the discharge (near the sharp edges) the field becomes weaker in the other part. To illustrate this I'll begin with the fact that all my high voltage experience gives the evidence that when the pulse is fast enough the breakdown voltage becomes independent of the electrodes shape. E.g. when You take a low inductance Marx bank and load it with the low ohm or low inductance shunt, and then when You make tests for sparks the length of those sparks is equal for ball electrodes and for needle ones. (Its seems rather obvious, since with nanosecond pulses there's no time for slow corona development or whatever. However I havent yet found a reference for this phenomenon in literature. It's probably too straightforward for authors to put on a red cloak of Captain The Obvious and to cry out loud about it. So here for this explanation I feel the need to refer to my own experience. For ones, who love the punctuality, I'll say that I'm not sure whether this phenomenon would stay right at too high field tensions, when a sufficient role is played by explosive autoemission from the cathode.) Further on if we take that the breakdown voltage really does not depend to the shape of the electrodes, it comes out that in the laser with sharp electrodes the most volume is filled with LOWER field than the average field in the laser with dull edges.

It even can be proven.

Let it be that in the first case the field is uniform and equal to ME (shortcut for the Medium Electricity):

------O MEMEMEME O------

If the inter electrode spacing is h and the voltage across the electrodes is U then U=h*ME

Let it be that in the second case the field is non uniform and equal to HE (High Electricity) in zone having radii r around the electrodes and equal to LE (Low Electricity) in the last area having the width h-2r :

------> HEHE) LELELE (HEHE <------

If the voltage across the electrodes is the same and equal to U, then U=HE*2r+LE*(h-2r)

If we make an equation from the expressions for the first case and for the second case we'll get:

h*ME=HE*2r+LE*(h-2r) => LE*(h-2r)=h*ME-HE*2r => LE=(h*ME-HE*2r)/(h-2r)
=> LE=(h*ME-2r*ME+2r*ME-HE*2r)/(h-2r)=>LE=(ME*(h-2r)+2r*ME-HE*2r)/(h-2r)
=> LE=ME+(2r*ME-HE*2r)/(h-2r) => LE=ME*[1+(2r-(HE/ME)*2r)/(h-2r)] =>
=> LE=ME*[1+2r*{1-(HE/ME)}/(h-2r)]

The expression in the curly braces is below zero, since HE>ME from the statement of problem. It means that in the square brackets the unity is summed with a negative number, and finally it means that LE<ME.

I.e. despite the fact that the field is strong near the sharp electrodes, the field in the last (and largest) part of the laser channel is always lower than the one in laser with the uniform field.

Ant the first glance it seems that we can only loose the overall laser output if we make the field strong in a small area and make it weak in much greater area. However it's not necessary true.

If we divide the working volume of the laser V into the volume occupied by the strong field Vhe and the volume occupied by weak field Vle (and V=Vhe+Vle) then we can express the output energy of the laser as:

W1=Vhe*eff(HE)*w(HE)+Vle*eff(LE)*w(LE)

where eff(HE) - laser efficiency with the strong field and eff(LE) - efficiency of the laser with the weak field, W(HE) - energy deposition in the area of the strong field, W(LE) - energy deposition in the area of the weak field.

The same is for the laser with the uniform field:

W0=V*eff(ME)*w(ME)

where eff(HE) - the laser efficiency for the medium field and W(ME) is the energy deposition in that medium field.

Now we can find a condition when the overall efficiency of the laser with non-uniform field is higher than the one for the laser with uniform field. For this we will assume that overall energy input is equal in both kinds of lasers:

V*w(ME)=Vhe*w(HE)+Vle*w(LE)

Lets look at the "addition" of the output energy of the non-uniform field laser in comparison to the uniform field one:

W1-W0=Vhe*eff(HE)*w(HE)+Vle*eff(LE)*w(LE)-V*eff(ME)*w(ME)=
=Vhe*eff(HE)*w(HE)+Vle*eff(LE)*w(LE)-eff(ME)*Vhe*w(HE)-eff(ME)*Vle*w(LE)=
=Vhe*w(HE)*[eff(HE)-eff(ME)] + Vle*w(LE)*[eff(LE)-eff(ME)]

In order for the effieiency of the non-uniform field laser be higher than the one of the uniform field laser this "addition" is needed to be positive: W1-W0>0, and thus:

Vhe*w(HE)*[eff(HE)-eff(ME)] + Vle*w(LE)*[eff(LE)-eff(ME)]>0 =>
=> Vhe*w(HE)*[eff(HE)-eff(ME)] > - Vle*w(LE)*[eff(LE)-eff(ME)] =>
=> Vhe*w(HE)*[eff(HE)-eff(ME)] > Vle*w(LE)*[eff(ME)-eff(LE)] =>

                 eff(HE)-eff(ME)   Vle*w(LE)
              => --------------- > ---------
                 eff(ME)-eff(LE)   Vhe*w(HE)

The translation of this condition from the math's to human language sounds like the requirement for the laser effectiveness function to grow so fast that the fraction in the left part of the inequality be higher than the right side (the latter does simply express the ratio of the energy deposited in the low field area to the energy deposited in the high field area).

It is not reasonable to expect that I will solve all the plasma-kinetic equations system, will get the explicit form of the eff(E) function and will prove the validity of the inequality gracefully. I just mean that in reality there may exist conditions, when the non uniform field laser has higher efficiency than the uniform field one. And those conditions take place when the efficiency grow strongly and non-linearly with the electric field growth (and it usually comes true).

One more notice. I understand that all these maths could be done using triple integrals from vector functions. However in order to illustrate the principles the school math is more than enough.

* Why the inter-electrode spacing is equal to the half of the one in the main spark gap?

Lets discuss a simple problem: a discharge of a capacitor through a zener diode (see the figure below)

At the initial moment let the capacitor Co be charged to the voltage Uo. Let the stabilization voltage of the Zener diode be U1 and let Uo>U1 (otherwise the current won't flow).

After the shortening of the switch some current i flows through the Zener diode. Thus the power deposited in the Zener diode is U1*i. The energy having been deposited in the Zener diode up to the full discharge of the capacitor is equal to the product of the current and voltage, integrated over the time. However U1 is constant and may be taken out of the integral.

It means that the total energy having been deposited in the Zener diode is equal to the product of U1 with the current integrated over the time. The latter is merely equal to the charge that have left the capacitor: Co(Uo-U1). (As You can see there's no need to know the current i and its time dependency.)

So far the total energy deposited in the Zener diode appeared to be equal toQ1=U1*Co(Uo-U1).

On the other hand the energy stored in the capacitor is Q0=Co*Uo^2/2.

The efficiency of the energy transfer from the capacitor to the zener diode is:

                   U1*Co(Uo-U1)   2*U1*(Uo-U1)
              eff= ------------ = ------------
                    Co*Uo^2/2         Uo^2

Using the school rules of the searching for extremum of a function (take the derivative and zero it) one may find that this efficiency is maximal (and equal to 50%) when the Uo=2*U1, i.e. when the charging voltage exceeds the voltage of stabilization twice.

What for we've discussed the problem of the zener diode? The reason is that with taking into account the "E/p ratio constantness for a gas discharge" the laser channel plays the role of zener diode in the problem solved just above. I.e. the efficiency of the energy transfer from the storage capacitor to the gas discharge plasma will be maximal when the storage capacitor is charged to the twice of the voltage of the discharge sustaining.

However our laser isn't a simple charge transfer type. It has a Blumlein circuit - the circuit, which is thought to double the voltage. Lets go further to a bit more complicated problem (see the picture below).

Now the zener diode not only included to a Blumlein circuit, but also connects to it not at the very beginning, but some time later, at the moment 'to'. At the beginning the right and left capacitors are charged to the voltage Uo. At this time the switch in the right capacitor's circuit becomes turned on, and the voltage on this capacitor UB begins to drop. Dependently to the relation of the RLC elements in this tank circuit the voltage may drop exponentially (if the decay is overcritical) or either by the cos(t/sqrt(LC)) law if the decay is subcritical.

At some moment of time (still prior to 'to') the voltage difference between left and right shoulders of the circuit reaches the laser channel breakdown voltage (or the stabilization voltage, that's equivalent). The gas discharge begins to evolve. Because it still evolves the electric current is still neglible and the switch in the zener diode circuit may be treated as open. Only after a time (namely the gas gap covering time) the discharge finishes its shape and begins to conduct - we may think that in our equivalent circuit the zener diode switch have turned to 'on' state - i.e. the 'to' moment of time have came.) Up to this moment the right capacitor has discharged some more, so the voltage difference between left and right capacitors would be not exactly equal to U1, but will be a bit higher: UA-UB=U1+deltaU

After that all processes are so fast that we can neglect further discharge of the right capacitor through the RLC circuit. Then all the process may be treated as the discharge of the left capacitor into the right one through the zener diode. If we designate the charge flown through the zener diode as q, we can express the voltages on the left and right capacitors as UA-q/C and UB+q/C. Apparently when the difference of those becomes less than stabilizing voltage U1 the current stops to flow and this condition may be used to calculate the flown electric charge:

UA-q/C-(UB+q/C)=U1 => q=(UA-UB-U1)(C/2)

(Actually in case of the real gas discharge the current usually does not stop after the voltage has dropped below its sustaining limit, it just contracts into "bright arc". But from the our pint of view it is invaluable since the further energy deposition is at least useless and may be not taken into account.)

Similar to the previous problem the usefull energy deposition in the zener diode is:

Q = q*U1 = U1*(UA-UB-U1)(C/2)

By variation of the spacing h in the laser channel we can vary the voltage of the discharge glow (the stabilization voltage) U1. Let's see what U1 is optimal (when UAand UB are given). If we take the derivative of Q (over U1) and nullify it, we'll get the condidtion of the maximum:

U1=(UA-UB)/2

If we assume that up to 'to' moment the left capacitor has kept all its charge (UA=Uo) and the right capacitor is fully discharged (UB=0) then the condition of the optimum will look like: U1=Uo/2 - i.e. breakdown voltage of the laser channel should be equal to a half of the charging voltage. And since the laser channel and the main spark gap are filled with the same air at the same pressure, it means that the interelectrode spacing in the laser channel should be the half of the interelectrode spacing in the main spark gap.

The last thing left to understand is why on the Earth the voltage on the right capacitor should be equal to zero? The deal is that the energy input depends not only to U1, but also to the UA-UB-U1 difference. The latter depends to how much charge will loose the right capacitor during the laser channel covering time. The rate of voltage change on the right capacitor is proportional to the current flowing in the RLC circuit: (dUB/dt)=iB/C, and this current is at top exactly when the voltage on the capacitor fly over zero (presuming the tank circuit is subcritical).

Kinda that, guys.

* The charge transfer circuit

One of the least tolerable drawbacks of the discussed above scheme of the laser (winged scheme aka voltage doubling circuit aka Blumlein circuit) is the necessity to replace the dielectric periodically. Can anybody somehow prolong its lifetime without lessening the output, i.e. without reducing the voltage and without reducing the energy input? It appears to be possible. It just needs the mylar to stay under the voltage as short time as it can. In the Blumlein laser the dielectric stays stressed during all the charging time. Ideally this charging time should be as short as possible, that means fast charging from some external capacitive storage.

Exactly this thing is done in so called "charge transfer circuit", that is shown on the picture below.

(Next to the laser channel (to the right) the grounded plate designates a preionizer)

The sharpening capacitor Cp (aka peaking capacitor, or, to be short, "the peaker") is usually made in the shape of a single plate having the length equal to the length of the laser electrodes and the width of 10..15 cm. Actually it strongly resembles a wing of Blumlein type laser. Charge transfer laser has only one wing. The second electrode is securely connected to the common wire (to the 'ground' plate). That design of the peaker provides the ability to discharge throughover that 1..2 nanoseconds needed for the ambient pressure nitrogen laser.

On the other hand the storage capacitor Cs has not to be as fast. In practice it is suitable to be made of commercial high voltage capacitors - all kinds of doorknob TDK's, Murata's, KVI-3's, or either made of (comparatively) low inductive film capacitors like Maxwells, K75-74 and so on. The commercial capacitors are usually reliable enough to provide the lifetime up to million pulses (if only the laser electrodes will endure this without need of the repolishing.)

One may use a DIY low ESL capacitor (how to make it see here). However it has low sense. Yes, such a capacitor can be made having even lower inductance than a commercial one has, but the lowest possible storage inductance is not the first requirement here. One comes to the charge transfer circuit exactly to lower the requirements to the storage by the price of the efficiency. And this allows to increase the lifetime. And it's sad to mention but it's very hard for DIYer to compete the commercial HV capacitors in the field of the lifetime.

Its obvious, that if the storage capacitor has not the lowest possible inductance, it has low sense to cut the inductance of the spark gap. So one can use here the most common spark gaps of the 'sphere-to-sphere' or the 'sphere-to-plane' types. The rail type multi-gapped spark gaps are too complex and may be avoided.

How it comes that without too much lowering of the inductance one can create a working nitrogen laser that often has even higher power? The figure below depicts two circuits.

A) - a charge transfer circuit
B) - the Blumlein scheme

The stray inductance of the circuit of the Blumlein wing reverse charging is designated as L2. The stray inductance of the charge transfer circuit is designated as L1. In order to understand how to reach the same or even higher power when L1>L2, let's calculate the usefull energy to gas input for both circuits. The 'usefull' energy input - means the one taking place during the time period shorter than that one-two nanoseconds, they always talk about.

A priori (i.e. without any proof) we'll assume that wings CL and CR of the Blumlein laser, as well as the wing Cp of the charge transfer laser are capable to discharge in the required time. Actually this assumption is trustworthy, as in both cases the wing is merely the planar transmission line, and the transmission lines are usually thought to be able to discharge in time equal to their (electrical) length divided by the speed of light.

In this case the usefull energy input for the Blumlein laser can be estimated as (see the previous part of the comments):

Qb=U1*(UL-UR-U1)(C/2)

and the one for the charge transfer laser:

Qct=U1*Cp*(Up-U1)

here C=CR=CL, U1 - the glow voltage for the discharge in the laser channel, Up - the voltage on the peaker Cp achieved up to the moment of the discharge ignition, UL-UR the difference of voltages on the wings of the Blumlein laser also achieved up to the moment of the ignition. It is clear that either Up or UL-UR are higher than the laser channel breakdown voltage exactly by the value of the voltage jump during the covering time tc.

The voltage jump can be estimated as the speed of the laser's wing charge (recharge) multiplied by the covering time. In its own turn the speed of charging/recharging may be estimated as the initial voltage divided by the discharge time. If we take a quarter of the oscillations period of the correspondent LC tank circuit as the discharge time, we can get:

for the Blumlein laser: UL-UR-U1=tc*Uob/[(pi/2)*sqrt(L2*C)]
for the charge transfer one: Up-U1=tc*Uoct/[(pi/2)*sqrt(L1*Cct)]

where Uob - the initial voltage of the Blumlein circuit, Uoct - the initial voltage of the charge transfer circuit, C - the Blumlein wing capacity (does not matter the right one or the left one since they are equal), Cct=Cs*Cp/(Cs+Cp) - the series capacity of the peaker with the storage (from the point of view of the current in the Cs-L1-Cp circuit they are connected in series).

One could also note that the recharging speed is nothing else than the current in the circuit divided by the capacity. From here it particularly follows that the energy input to the gas is at the maximum (when all other conditions are fixed) if the ignition of the laser channel takes place at the moment of maximum of the current in the tank circuit. And that maximal current might be estimated a bit more precisely using the energy
preservation law (C*U^2/2=L*I^2/2), but this approach will give us the relation between the charging voltage and the breakdown voltage. And we don't need it right now since we're searching when the charge transfer laser has higher energy deposition regardless to its efficiency.

With knowing the voltage jump during the covering time we can now write the usefull energy depositions:

for the Blumlein laser:

Qb=U1*(UL-UR-U1)(C/2)=U1*(C/2)tc*Uob/[(pi/2)*sqrt(L2*C)]

for the charge transfer laser:

Qct=U1*Cp*(Up-U1)=U1*Cp*tc*Uoct/[(pi/2)*sqrt(L1*Cct)]

Now let's look when it can take place that Qct > Qb, i.e:

U1*Cp*tc*Uoct/[(pi/2)*sqrt(L1*Cct)] > U1*(C/2)tc*Uob/[(pi/2)*sqrt(L2*C)]

If both lasers have the same spacing in their laser channels, the glow voltage and the covering time will be the same. So we can reduce the expression by U1 and tc. In addition let's remove the (pi/2) too:

Cp*Uoct/sqrt(L1*Cct) > (C/2)*Uob/sqrt(L2*C) =>

Cp^2*Uoct^2/(L1*Cct) > (C^2/4)*Uob^2/(L2*C) =>

Cp^2*Cs*Uoct^2/(L1*Cs*Cct) > 2*C*Uob^2/(8*L2) =>

Cp^2*Eoct/(L1*Cs*Cct) > Eob/(8*L2)

where Eoct=Cs*Uoct^2/2 - the initial energy stored in the charge transfer laser and Eb=2*C*Uob^2/2 - is the initial energy stored in the Blumlein laser wings.

Eoct/Eob > (L1*Cs*Cct)/(8*L2*Cp^2) =>

Eoct/Eob > (L1*Cs*Cs*Cp)/(8*L2*Cp^2*(Cp+Cs)) =>

Eoct/Eob > (L1*Cs*Cs)/(8*L2*Cp*(Cp+Cs)) => ...

Eoct/Eob > (1/8)*(L1/L2)*(Cs/Cp)/(1+Cp/Cs)

Now the left part consists of the charge transfer laser's stored energy divided by the stored energy of the Blumlein laser. The left part keeps the inductance of the charge transfer laser charging circuit divided to the inductance of the Blumlein laser reverse circuit, and this ratio is multiplied by some factor, that depends to the relation between the peaker and storage capacities.

One can now see that the slowness of the laser with the higher inductance may be compensated by the higher initial energy stock. This way one can achieve high output energy of the charge transfer lasers by loosing the efficiency.

One can go even further and assume that Cp=C (it means that, as it oftenly happens, the sizes and shape of the Blumlein laser wing are similar to the ones of the charge transfer laser wing). In this case if in our final expression we return from the energies to the "C*U^2" terms, if we note, that in most cases Cs>>Cp and therefore (1+Cp/Cs)->1, then with taking into account all this stuff we'll get:

Cs*Uoct^2/[2*C*Uob^2] > (1/8)*(L1/L2)*(Cs/Cp)/(1+Cp/Cs) =>

(Cs/Cp)*Uoct^2/Uob^2 > (1/4)*(L1/L2)*(Cs/Cp)/(1+Cp/Cs) =>

Uoct^2/Uob^2 > (1/4)*(L1/L2)/(1+Cp/Cs) =>

Uoct/Uob >~ (1/2)*sqrt(L1/L2)

Now one can see that the main resource for the mentioned above increasement of the energy, needed to compensate higher inductance, lies not in the increasing of the storage capacity Cs, but in the increasement of the power supply voltage Uct. It finely correlates the typical design of the charge transfer laser: large and very high voltage rated storage capacitor (preferably of the commercial type), that uses a common (pinpoint type) spark gap to charge a peaking capacitor having the shape of a metal foil wing placed above a leaf of thin dielectric. One of the laser electrodes is securely connected to this wing all over its length. The other laser electrode is similarly connected to the common wire ("ground" plate). It is obvious that the voltage on the peaking capacitor does never exceeds the breakdown voltage of the laser channel by any significant value. This allows to design the peaker to be rather low voltage rated, i.e. having thin dielectric, so its specific capacity can be high enough.

Here is the photo of a charge transfer circuit laser.

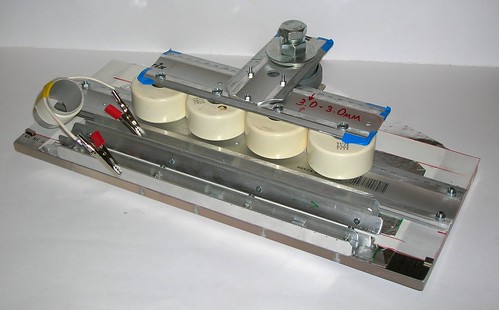
Four 2 nf 40 Kv rated Murata's serve as the storage capacitor. The spark gap is of the "ball-against-plane" type. The peaker wing is sized as 30x10 cm. The dielectric is a mylar sheet 0.12 mm thick. When the spacing in the spark gap is as hig as 12 mm the output energy of this laser does slightly exceed the output of the Blumlein laser described above. The size and shape of the electrodes are the same in both types of lasers. The optimal spacing in the laser channel is higher than the one in the Blumlein laser by 0.2..0.3 mm.

Due to the higher initial voltage and higher stored energy the charge transfer laser has the repetition rate nearly 10 times lower than the one for the Blumlein type laser when fed from the equivalent power supply. In almost 2 years of operation its dielectric has never failed. However it does not help to get rid of the periodic electrodes cleaning. This problem arises here even more sharply than in the winged laser. There essentially exists a problem of an excessive energy deposition.

In the Blumlein type laser the wings come to the voltage equilibrium in a short time and the energy deposition to gas stops. But in the charge transfer laser, even when the peaker has dropped its excessive charge throughover the laser channel, it stays connected to the powerfull and slow discharging storage capacitor Cs. The gas discharge continues to glow (or burn?) until the total Cs+Cp capacity drops its voltage to the low enough values. I.e. the total energy deposition (the sum of the fast one and slow one) will be by the next value higher:

X = Cs*U1*(Uoct-U1)/{U1*Cp*tc*Uoct/[(pi/2)*sqrt(L1*Cct)]} =>
X = Cs*(Uoct-U1)/{Cp*tc*Uoct/[(pi/2)*sqrt(L1*Cct)]}

If we neglect the laser channel breakdown voltage in comparison with the initial voltage (i.e. if we take that Uoct-U1 ~ Uoct, it means that we consider the 3 mm of the laser channel spacing to be neglible when compared to the 12 mm of the main spark gap spacing), then:

X = Cs/{Cp*tc/[(pi/2)*sqrt(L1*Cct)]} =>
X = (Cs/Cp)*{(pi/2)*sqrt(L1*Cct)/tc}

The value on the right side of the expression is the ratio of the storage capacity to the peaker capacity multiplied by the ratio of the storage discharge time to the covering time. Usually both of these factors are high enough to securely provide the excess of the energy deposition above the threshold of the discharge contraction. In its own turn this leads to the accelerated erosion of the laser electrodes.

Here comes one curious conclusion: in order for not to heat the gas in excess, the storage capacity should be LOWER than the peaker capacity. Evidently the voltage on the storage should be raised correspondently (and it is not always easy to do). When the storage voltage is large enough the storage itself may be better designed as a Marx bank generator, and this design we can see in the most advanced nitrogen lasers.

One more notice to the end of this part: here it was always assumed that the covering time is always less than the typical time of the laser wings charging or the charging time of the peaking capacitor. Otherwise the above formulas arent correct. However this condition fulfillment is trustworthy for the ambient pressure nitrogen lasers. From the difference of the spacings between the laser electrodes at both ends of the laser when the slope of the electrodes is optimal (0.2..0.3 mm per 30 cm of length) we can estimate the covering speed as high as 0.2..0.3 mm/ns, and therefore the covering time will be ~10 ns. On the other hand if we estimate the discharge circuit inductance to be ~20 nH, then the wing voltage reversal time will be pi*sqrt(LC)= 3.14*sqrt(6nF*20nH)=34ns. For the charge transfer laser this time will be even higher for the obvious reasons. However if we take that the covering speed depends only to the electron velocity (read this as E/p ratio), i.e. it practically does not depend to the pressure, then in low pressure nitrogen lasers with their huge spacings (up to 10..30 mm) this condition may appear to be invalid.

The value of 0.3 mm/ns taken from the difference of spacings in 30 cm long laser may seem to be doubtful for some extent. Especially if one takes a look into the graphs given in Bergmann’s article devoted to his microlasers. The current to voltage delay there appears to be clearly 2 ns for 2 mm of spacing, so the covering speed seems to be equal 1mm/ns – the value given in most textbooks, and also the one I used earlier. This one looks more trustworthy but requires the explanation why the optimal difference of the electrodes spacing is less than 1 mm per each 30 cm of the laser channel length. The case may be due to the discharge instabilities at higher spacings.

Its up to You what value of the covering speed to use (0.3 mm/ns or 1 mm/ns), it only affects the resulting digits but does not change the tendencies.

* Gas mixture

Despite the fact that the laser was initially intended to be capable for operation with air only (and without all that gas tank husbandry and other excesses) it was interesting how much output can it give if working with technical grade nitrogen.

A special lid was developed to cover the laser channel. It has a special hose and allows to use the laser while it is being blown through by the gas mixture from an external vessel (from a gas cylinder or an automotive tyre filled with the premixed gases).

It was found out that when the laser is being continuously blown by the nitrogen, the dye cells are able to lase at a distance three times greater than during normal operation. With taking into account the "inversed square of r" law it means that the laser's power rises almost by ten times. The nitrogen laser's spot and the spots of dye lasers driven by it have strongly improved their visual brightness too.

When the working gas is the common air with its oxygen having been burned out by combusting organic fuel (so called "combustor" gas) the laser works almost as effectively as with air. (I.e. "combustor" does not improve anything".)

Tests on the helium with air mixture have shown that the power of the laser begins to rise when there is less than 20% of air in the mixture. The mixture containing air:helium = 1:10 (by volume) the power is comparable to the one with pure nitrogen (or to be more precise the dye cells lase at similar distances. The power/energy can only be treated as "the same" if the pulse duration does not vary here.)

For the reasons mentioned above it is very interesting to test the laser with N2:SF6 and N2:SO2 mixtures. However since the laser is not vacuum tight the exhaust of the sulfur dioxide into the air would require very vell vetntilated premises. And I have no idea where to get a tank of SF6.

* Scaling

It is usually assumed that since the excited nitrogen lifetime is very small at atmospheric pressure, the length of the laser should not exceed a certain value. The limiting length is commonly taken as long as 30 cm - the distance that light travels in a single nanosecond.Actually the same phenomenon (the traveling wave of the electric discharge ignition), that allows You to tune the laser for the maximum light in the desired direction only by slanting the electrodes, does also allow to synchronize the excitation with the traveling light over the very significant lengths. At least up to meter (tested) or may be several meters (untested). I.e. the laser can e easily scaled by length. If the length is reasonable (longer than 10..15 cm) the power grows linearly with the length of the laser. However the excessive factor of two or three in the laser's output gives a very little addition to its possibilities.
On the other hand, such a large thing as a meter long laser on Your table will definitely not make You happy.

If trusting the scientific papers, the laser can be easily scaled towards diminishing of length. One should keep in mind that the "threshold" length is somewhere between 5 and 10 cm and one should use resonators and mirrors if the laser becomes so small. (I know, i know, the superradiance (aka ASE) is the phenomenon without the threshold. However at some point the output anyways begins to degrade dramatically.)

In theory the laser should be easily scalable to the width with the corresponding increasement of the feeding voltage. In practice for the transversal discharge in the air three millimeters is a kinda magical number. Below it the volumetric discharge can be created rather easily, and above it the difficulties rise catastrophically. In principle I was able to create a nitrogen laser grade volumetric discharge with a width of 5(!) mm. however it required to increase the feeding voltage by 4 times, to increase the voltage rise rate more than by an order of magnitude, to completely rework the pulse forming circuit (to use a stripline Marx bank if anyone is interested into) and to rework the preionization technique. The power of laser did also rise, however the result was definitely not worth its expenses and difficulties.

The scaling to the thickness will force You to go away from the sharp electrodes to something like rounded planar shape. However instead of the expected increasement of the working volume and power it will just cause the drop of the efficiency and thus the drop of the laser output. So there are no much of the reserves in that way too.

* The POWER (Honest and Shameless)

The story about the power I really dunno where to start from.

Maybe I should say that there was at leas one commercial (and tested) calorimeter that gave the reading of 60 mW when lit with this laser and at the same time my homemade Peltier calorimeter shows zero in the limits of measurement errors. Need to say that both of the measurers demonstrate the amazing accordance when they measure the power of some laser pointer.

Or either I could start from the fact that once upon a time I've seen people, who used a pyroelectric OPHIR measurer, specially intended for use in microjoules/milliwatts range, and they used this device to measure the power of way too weaker nitrogen laser than the described above. They measured as much as 5 mW, while that laser could not drive any dyes to lase even when equipped with a good lense.

Or maybe I should start from that the people having megawatt (at least rated so) nitrogen lasers, when see what does the nitrogen laser described in this guide, do typically begin their speech with the words "So damned much..."

When I say that the homemade Peltier calorimeters shows nothing it's actually not all the truth. It actually shows much... more than it should. As it was already told, the nitrogen laser is a powerfull source of the electromagnetic interference. Many devices go mad near it and the Peltier calorimeter is not the happy exclusion. One should probably say that the measurements are just impossible here. However the things are slightly better. If You have ever measured a laser pointer power with the Peltier calorimeter, You could note that after the laser was turned off the gauge's readings do not instantly become zero. They gradually decrease - the device slowly looses its accumulated heat. Using the readings in the process of the device cooling one can discover what were the readings when the laser was turned on. To do this correctly one should measure the time dependence of the readings during the cooling. Then one should draw a graph in logarithmic scale and draw an approximation line, and prolong it to the origin of the coordinates... Or one can make a microchip circuit intended to do all these things automatically. However if we speak not about the precise measurements, but about the question: "zero or not the zero?" all those actions look a bit excessive. If the laser gave some measurable power when it was turned on, one should be able to clearly see how the gauge readings decrease. And here is nothing like that. The laser is turned off, there is no electromagnetic interference, and nothing seems to interfere with measurements. But the calorimeter behaves like it has never been heated to any measurable extent.

Worth to mention another phenomenon. The powerfull interference do somehow affect the measurer circuit. It may be that the automatic gain control becomes overloaded. Or some field transistor collects a charge on its gate. Anyways it looks like the readings stick to some value (particurlarly to zero) and stay at this value for some time after the source of the interference was turned off. It is clear that after ten-fifteen seconds of waiting the residual readings are not informative. Fortunately this phenomenon appears to be easy to suppress by shunting the input terminals by a low inductance capacitor and resistor. After this modification the dead time of the measurer comes close to zero. Apparently thoroughful and careful shielding and grounding can zero any unwanted interference, however it only sounds simple. Wrapping the devices into aluminium foil does amazingly give nothing - the ultra high frequency pickup - escapes though any leakages. And erection of a vacuum tight metal box with thick walls is a long history, not too easy and rather expensive too.

Finally when You've got rid of the interference (or found a way how to do Your nifty work under their affection), its way not the end. Rather then to describe all possible causes of the mistakes, I'll use an example from my own experience. Exactly the laser described here was the first pretendent to be one that is capable to make sufficient readings on the interference protected Peltier calorimeter. Everything seemed to be good: When I blocked the beam path with a piece of paper it showed zero, when I opened the beam path the readings were inplace. Once it happened to me to forget an empty dye laser cuvette on the way of the beam. When I turned the laser on I was discouraged by the fact that the indicated power was zero. Can the glass absorb the nitrogen laser's wavelength so strongly? If it could the dye won't lase. Or either it would not lase if another thin glass plate was inserted between it and the nitrogen laser. So the problem was not in glass. Later I found out that if the beam path was blocked by some transparent thing (glass, mica plexiglass, mylar) the power meter readings dropped from certain measurable values to definitely immeasureable ones. It means something else than the (UV) light carries the heat from laser to calorimeter. Most probably it was the air having been heated in the discharge. The air in the discharge suffers from the sufficient heating. One may prove it by calculations and by the emitted light and sound. And the laser channel itself represents a well formed barrel that shoots the hot air jet towards where it is directed. Even more curious that this jet has low divergency and affects the calorimeter at the distances up to a meter. The details of the process are unclear to me. It reminds vortex rings, that can travel a long way without any decay. Actually the details are of no importance here. Of the most importance is the fact that the measurements that seemed to be trustworthy appeared to give completely wrong result. (Another variant of explanation is a proposition that the laser emits some radiation away from the glass transparency range. E.g. somewhere near 5 or 6 mcm. However this is completely unlikely to be true.)

I should also note that the measurements are usually conducted in correspondence to the expectations. If the meter shows something You are awaiting for, You write down the result and trust in it. If otherwise the device shows something unexpected, You start to look for a mistake. The funny thing is that in this particular case the expected result is the wrong one.

I think that after all said above it should be evident that simply to lit the eye of the calorimeter by the laser is the direct way to error. And in most cases the measurements are conducted exactly that way. Its a pity, but oftenly one becomes aware about that only after the measurements were performed, published and even forgotten. Maybe after years have passed. I am not an exclusion myself. The example is my own guide on the low power TEA nitrogen laser where a noble figure of 5 mW was estated. Lol at me. Now I already dont believe in any measurements of milliwatts and microjoules. If the measurements can not be approved by making a hole in a (carbon) paper I dont trust them. In order for not to repeat the previous mistakes I will abstain from naming certain numbers. However the Alfonso Torres Rodrigues estimation of power of a similar laser, saying ~50 kW (~50 mcJ) looks rather adequate. (Maybe minus an order of magnitude.)

And if the 50 mcJ ranked nitrogen laser with its unfocused beam can drive the best dyes to lasing at distances of above 1 meter. Just imagine what can do a millijoule rated nitrogen laser? And the 10-20 mJ one? I won't be surprised too much if the concrete wall will start to lase under such a beam.

<< HOME PAGE